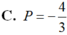a) \(sin^2\widehat{AMB}=1-cos^2\widehat{AMB}\)
\(\Rightarrow sin^2\widehat{AMB}=1-\dfrac{25.13}{26^2}=\dfrac{351}{26}\)
\(\Rightarrow sin\widehat{AMB}=\dfrac{3\sqrt{39}}{26}\)
\(\dfrac{AB}{sin\widehat{AMB}}=2R_{ABM}\Rightarrow R_{ABM}=\dfrac{AB}{2sin\widehat{AMB}}=\dfrac{3}{2.\dfrac{3\sqrt{39}}{26}}=\dfrac{\sqrt{13}}{\sqrt{3}}\ne\sqrt{2}\)
Nên Câu A sai
b) \(cos\widehat{AMC}=cos\left(180^o-\widehat{AMB}\right)=-cos\widehat{AMB}=-\dfrac{5\sqrt{13}}{26}\)
Nên Câu B sai
c. Đúng
Đặt \(AM=x\)
Áp dụng định lý hàm cosin trong tam giác ABM:
\(AB^2=BM^2+AM^2-2BM.AM.cos\widehat{AMB}\)
\(\Leftrightarrow3^2=4^2+x^2-8x.\dfrac{5\sqrt{13}}{26}\)
\(\Rightarrow x^2-\dfrac{20\sqrt{13}}{13}x+7=0\Rightarrow\left[{}\begin{matrix}x=\sqrt{13}\\x=\dfrac{7\sqrt{13}}{13}\end{matrix}\right.\)
d. Đúng
Do A nhọn và A lớn nhất trong 3 góc nên \(60^0< A< 90^0\) \(\Rightarrow cosA>0\)
\(\Rightarrow cos\widehat{BAM}>0\) do \(\widehat{A}>\widehat{BAM}\)
Nếu \(AM=\dfrac{7\sqrt{13}}{13}\), áp dụng định lý hàm cos trong tam giác ABM:
\(\Rightarrow cos\widehat{BAM}=\dfrac{AB^2+AM^2-BM^2}{2AB.AM}=\dfrac{3^2+\left(\dfrac{7\sqrt{13}}{13}\right)^2-4^2}{2.3.\dfrac{7\sqrt{13}}{13}}=-\dfrac{\sqrt{13}}{13}< 0\) (ktm)
\(\Rightarrow AM=\sqrt{13}\)
Khi đó: \(cosB=\dfrac{AB^2+BM^2-AM^2}{2AB.BM}=\dfrac{1}{2}\)
\(\Rightarrow AC=\sqrt{AB^2+BC^2-2AB.BC.cosB}=7\)