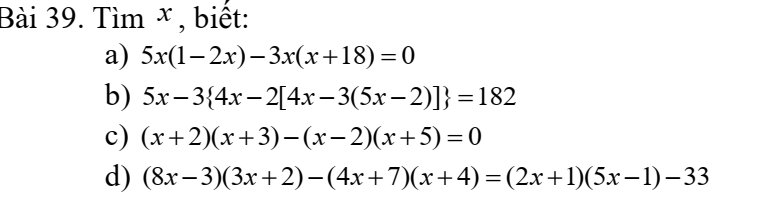a: \(5x\left(1-2x\right)-3x\left(x+18\right)=0\)
=>\(5x-10x^2-3x^2-54x=0\)
=>\(-13x^2-49x=0\)
=>x(13x+49)=0
=>\(\left[{}\begin{matrix}x=0\\13x+49=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-\dfrac{49}{13}\end{matrix}\right.\)
b: \(5x-3\left\{4x-2\left[4x-3\left(5x-2\right)\right]\right\}=182\)
=>\(5x-3\left\{4x-2\left[4x-15x+6\right]\right\}=182\)
=>\(5x-3\left\{4x-2\cdot\left(-11x+6\right)\right\}=182\)
=>\(5x-3\left\{4x+22x-12\right\}=182\)
=>\(5x-3\left(26x-12\right)=182\)
=>5x-78x+36=182
=>-73x=182-36=146
=>x=-2
c: \(\left(x+2\right)\left(x+3\right)-\left(x-2\right)\left(x+5\right)=0\)
=>\(x^2+5x+6-\left(x^2+3x-10\right)=0\)
=>\(x^2+5x+6-x^2-3x+10=0\)
=>2x+16=0
=>2x=-16
=>x=-8
d: \(\left(8x-3\right)\left(3x+2\right)-\left(4x+7\right)\left(x+4\right)=\left(2x+1\right)\left(5x-1\right)-33\)
=>\(24x^2+16x-9x-6-\left(4x^2+16x+7x+28\right)=10x^2-2x+5x-1-33\)
=>\(24x^2+7x-6-4x^2-23x-28=10x^2+3x-34\)
=>\(20x^2-16x-10x^2-3x=0\)
=>\(10x^2-19x=0\)
=>x(10x-19)=0
=>\(\left[{}\begin{matrix}x=0\\x=\dfrac{19}{10}\end{matrix}\right.\)
a) \(5x\left(1-2x\right)-3x\left(x+18\right)=0\Rightarrow5x-10x^2-3x^2-54x=0\Rightarrow-13x^2-49x=0\Rightarrow-x\left(13x+49\right)=0\Rightarrow\left[{}\begin{matrix}-x=0\\13x+49=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{-49}{13}\end{matrix}\right.\)
Vậy x=0; x=-49/13
b) \(5x-3\left\{4x-2\left[4x-3\left(5x-2\right)\right]\right\}=182\Rightarrow5x-3\left[4x-2\left(4x-15x+6\right)\right]=182\Rightarrow5x-3\left[4x-2\left(-11x+6\right)\right]=182\Rightarrow5x-3\left(4x+22x-12\right)=182\Rightarrow5x-3\left(26x-12\right)=182\Rightarrow5x-78x+36=182\Rightarrow-73x=146\Rightarrow x=-2\)
Vậy x=-2
c) \(\left(x+2\right)\left(x+3\right)-\left(x-2\right)\left(x+5\right)=0\Rightarrow x^2+5x+6-\left(x^2+3x-10\right)=0\Rightarrow x^2+5x+6-x^2-3x-10=0\Rightarrow2x=4\Rightarrow x=2\)
Vậy x=2
d) \(\left(8x-3\right)\left(3x+2\right)-\left(4x+7\right)\left(x+4\right)=\left(2x+1\right)\left(5x-1\right)-33\Rightarrow24x^2+16x-9x-6-\left(4x^2+16x+7x+28\right)=10x^2-2x+5x-1-33\Rightarrow24x^2+7x-6-4x^2-23x-28=10x^2+3x-34\Rightarrow10x^2-19x=0\Rightarrow x\left(10x-19\right)=0\Rightarrow\left[{}\begin{matrix}x=0\\10x-19=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{19}{10}\end{matrix}\right.\)
Vậy x=0; x=19/10