Question

Answer 1

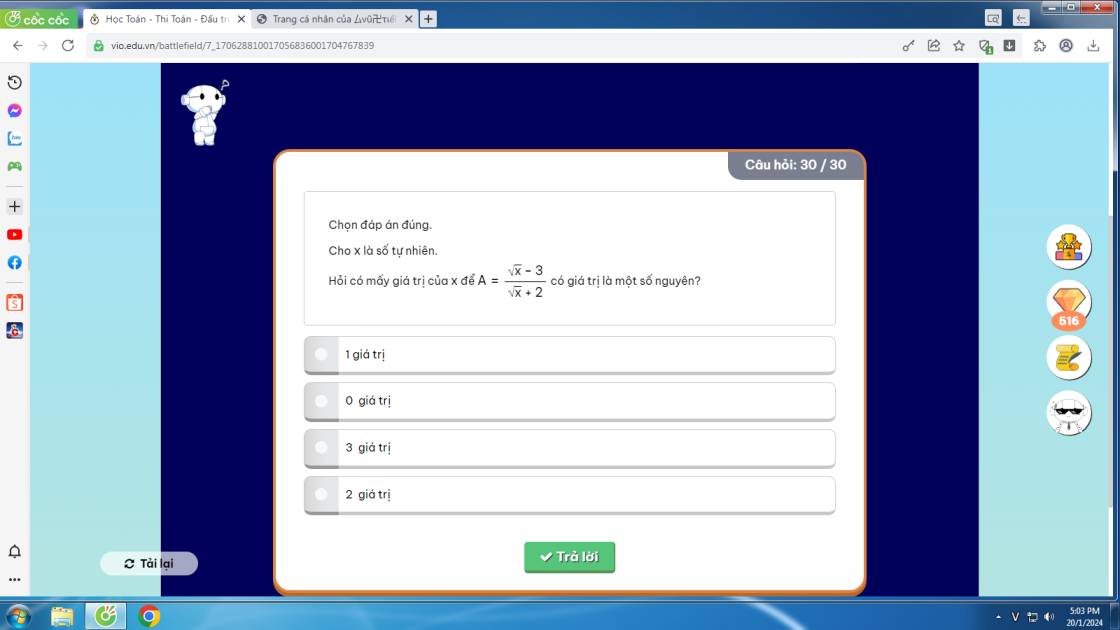
Ta có: \(A=\dfrac{\sqrt{x}-3}{\sqrt{x}+2}=\dfrac{\sqrt{x}+2-5}{\sqrt{x}+2}=1-\dfrac{5}{\sqrt{x}+2}\left(x\ge0\right)\)

Để \(A\) có giá trị nguyên thì \(\dfrac{5}{\sqrt{x}+2}\) có giá trị nguyên

\(\Rightarrow5⋮\sqrt{x}+2\)

\(\Rightarrow\sqrt{x}+2\inƯ\left(5\right)\)

\(\Rightarrow\sqrt{x}+2\in\left\{1;5;-1;-5\right\}\)

Mà \(\sqrt{x}+2\ge2\forall x\ge0\)

nên \(\sqrt{x}+2=5\Leftrightarrow\sqrt{x}=3\)

\(\Rightarrow x=9\left(tm\right)\)

Vậy có 1 giá trị của x thoả mãn A nguyên.