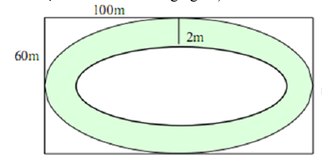
Đặt \(x=2\tan t\Rightarrow dx=\frac{2}{\cos^2t}dt\)
\(x=0\Rightarrow t=0,x=1\Rightarrow t=\arctan\dfrac{1}{2}\)
\(I=\int\limits_0^{\arctan\frac{1}{2}}\sqrt{4\tan^2t+4}.\dfrac{2}{\cos^2t}dt=\int\limits_0^{\arctan\frac{1}{2}}\dfrac{4}{\cos^3t}dt\)
Đặt \(u=\sin t\Rightarrow du=\cos tdt\)
\(t=0\Rightarrow u=0,t=\arctan\dfrac{1}{2}\Rightarrow \tan t=\dfrac{1}{2},\cos t=\dfrac{1}{\sqrt{1+\tan^2 t}}=\dfrac{2}{\sqrt{5}}\Rightarrow u=\sin t=\dfrac{1}{\sqrt{5}}\)
\(I=\int\limits_0^{1/\sqrt{5}}\dfrac{4}{(1-u^2)^2}du=\int\limits_0^{1/\sqrt{5}}\left(\dfrac{1}{u-1}-\dfrac{1}{u+1}\right)^2du=\int\limits_0^{1/\sqrt{5}}\left(\dfrac{1}{(u-1)^2}+\dfrac{1}{(u+1)^2}-\dfrac{2}{(u-1)(u+1}\right)du\)
\(\Rightarrow I=\int\limits_0^{1/\sqrt{5}}\left(\dfrac{1}{(u-1)^2}+\dfrac{1}{(u+1)^2}-\dfrac{1}{u-1}+\dfrac{1}{u+1}\right)=\left(-\dfrac{1}{u-1}-\dfrac{1}{u+1}-\ln|u-1|+\ln|u+1|\right)\Big|_0^{1/\sqrt{5}}\)