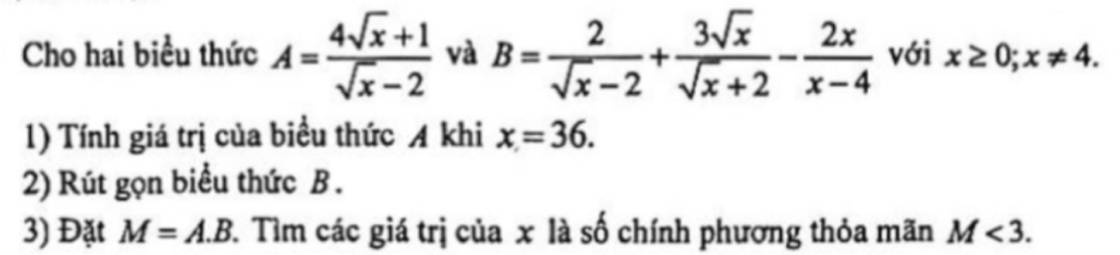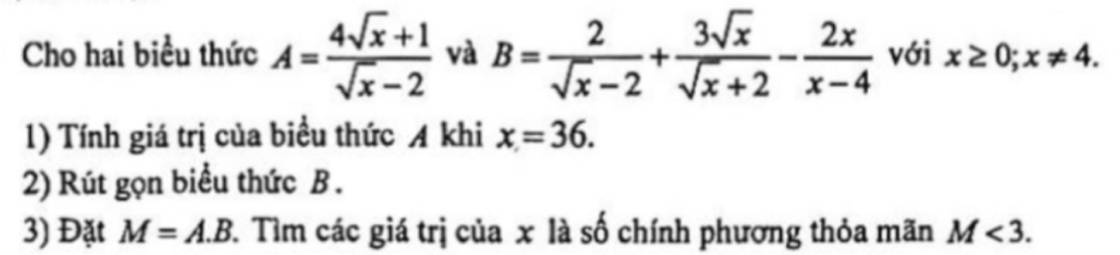1) Thay x=36 vào ta có:
\(A=\dfrac{4\cdot\sqrt{36}+1}{\sqrt{36}-2}=\dfrac{4\cdot6+1}{6-2}=\dfrac{25}{4}\)
2) \(B=\dfrac{2}{\sqrt{x}-2}+\dfrac{3\sqrt{x}}{\sqrt{x}+2}-\dfrac{2x}{x-4}\)
\(B=\dfrac{2}{\sqrt{x}-2}+\dfrac{3\sqrt{x}}{\sqrt{x}+2}-\dfrac{2x}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-3\right)}\)
\(B=\dfrac{2\left(\sqrt{x}+2\right)}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}+\dfrac{3\sqrt{x}\left(\sqrt{x}-2\right)}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}-\dfrac{2x}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}\)
\(B=\dfrac{2\sqrt{x}+4+3x-6\sqrt{x}-2x}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}\)
\(B=\dfrac{x-4\sqrt{x}+4}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}\)
\(B=\dfrac{\left(\sqrt{x}-2\right)^2}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}\)
\(B=\dfrac{\sqrt{x}-2}{\sqrt{x}+2}\)
3) \(M=A\cdot B=\dfrac{4\sqrt{x}+1}{\sqrt{x}-2}\cdot\dfrac{\sqrt{x}-2}{\sqrt{x}+2}\)
\(M=\dfrac{4\sqrt{x}+1}{\sqrt{x}+2}\)
Ta có: \(M< 3\) khi và chỉ khi:
\(\dfrac{4\sqrt{x}+1}{\sqrt{x}+2}< 3\) (ĐK: x là số chính phương)
\(\Leftrightarrow\dfrac{4\sqrt{x}+1-3\left(\sqrt{x}+2\right)}{\sqrt{x}+2}< 0\)
\(\Leftrightarrow\dfrac{4\sqrt{x}+1-3\sqrt{x}-6}{\sqrt{x}+2}< 0\)
\(\Leftrightarrow\dfrac{\sqrt{x}-5}{\sqrt{x}+2}< 0\)
Mà: \(\sqrt{x}+2\ge2>0\forall x\)
\(\Leftrightarrow\sqrt{x}-5< 0\)
\(\Leftrightarrow\sqrt{x}< 5\)
\(\Leftrightarrow x< 25\)
Nên: \(x\in\left\{0;1;4;9;16\right\}\)
làm hộ em phần 3) thôi ạ mấy phần kia em đã làm đc r ạ
