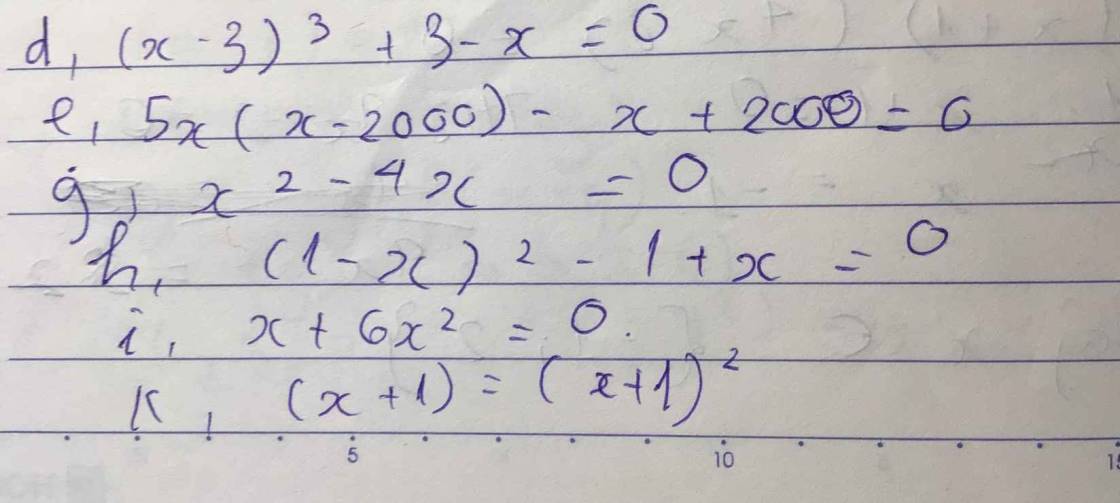Câu 3:
a: ĐKXĐ: \(x\notin\left\{2;-2\right\}\)
b: \(A=\dfrac{\left(x+2\right)^2}{\left(x+2\right)\left(x-2\right)}=\dfrac{x+2}{x-2}\)
Câu 1:
a: \(\left(x-3\right)^2=x^2-6x+9\)
bL \(x^2-y^2=\left(x-y\right)\left(x+y\right)\)
câu 6 :
Q = \(\dfrac{2x^2+2}{\left(x+1\right)^2}\)
Đặt x + 1 = t
=> x = t - 1
\(\Rightarrow Q=\dfrac{2\left(t-1\right)^2+2}{t^2}\)
\(\Rightarrow Q=\dfrac{2t^2-4t+2+2}{t^2}\)
\(\Rightarrow Q=\dfrac{2t^2-4t+4}{t^2}=\dfrac{2t^2}{t^2}-\dfrac{4t}{t^2}+\dfrac{4}{t^2}\)
\(\Rightarrow Q=2-\dfrac{4}{t}+\dfrac{4}{t^2}=1+\left(1-\dfrac{4}{t}+\dfrac{4}{t^2}\right)\)
\(\Rightarrow Q=1+\left(1-\dfrac{2}{t}\right)^2\ge1\) Vì \(\left(1-\dfrac{2}{t}\right)^2\ge0\)
Vậy GTNN của Q là 1
Dấu = xảy ra :\(\Leftrightarrow\left(1-\dfrac{2}{t}\right)^2=0\Leftrightarrow\left(1-\dfrac{2}{x+1}\right)=0\Leftrightarrow\dfrac{2}{x+1}=1\Leftrightarrow x+1=2\Leftrightarrow x=1\)
Câu 4 :
Xét tứ giác ABCD có : \(\widehat{A}+\widehat{B}+\widehat{C}+\widehat{D}=360\)
=> x + 130 + 90 + 70 = 360
=.> x = 70
Vậy x = 70

 mọi người bít làm câu nào chỉ giúp với,cần rất gấp:<
mọi người bít làm câu nào chỉ giúp với,cần rất gấp:<