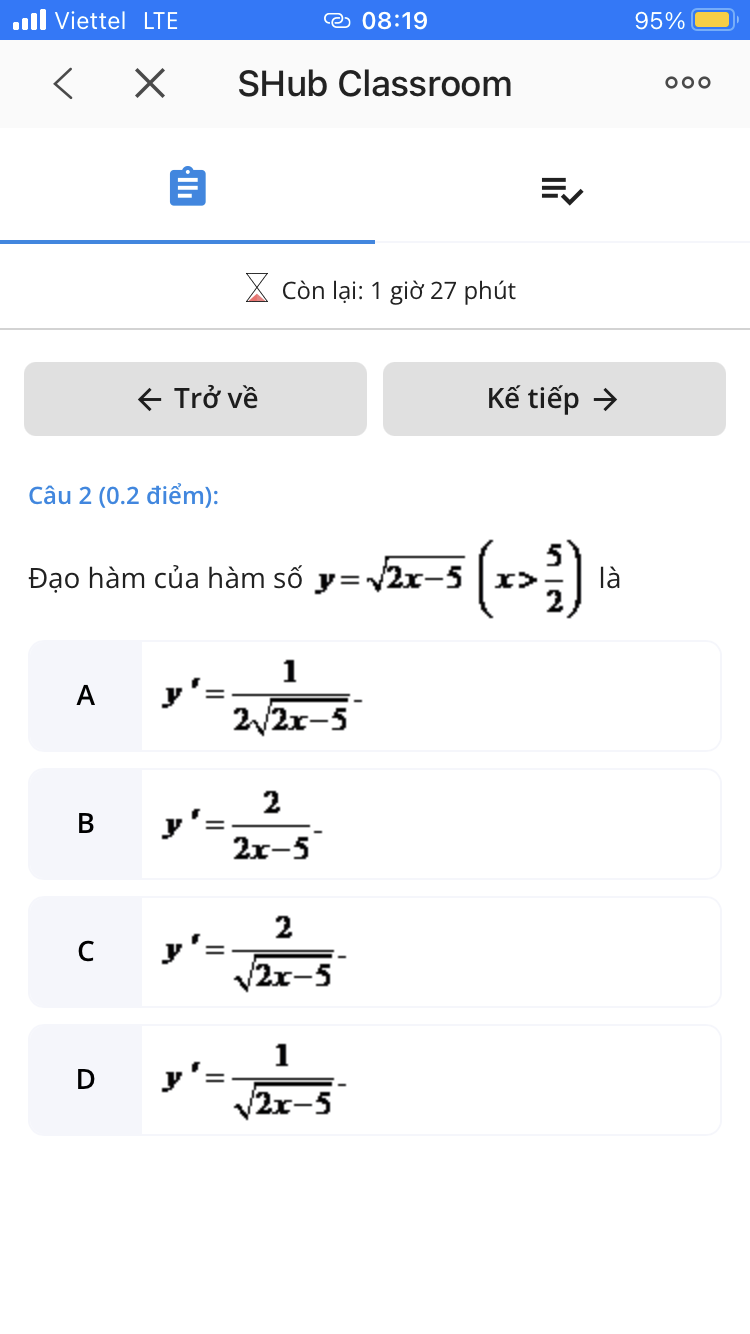
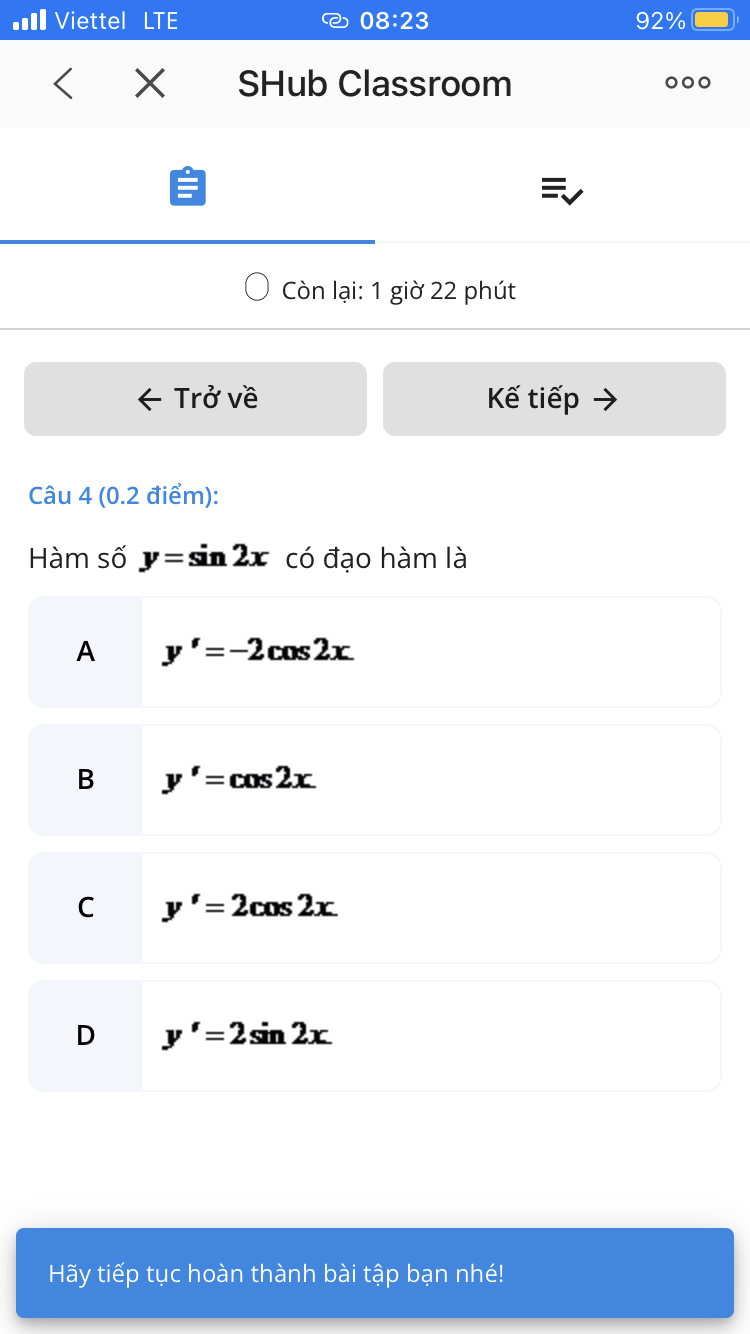
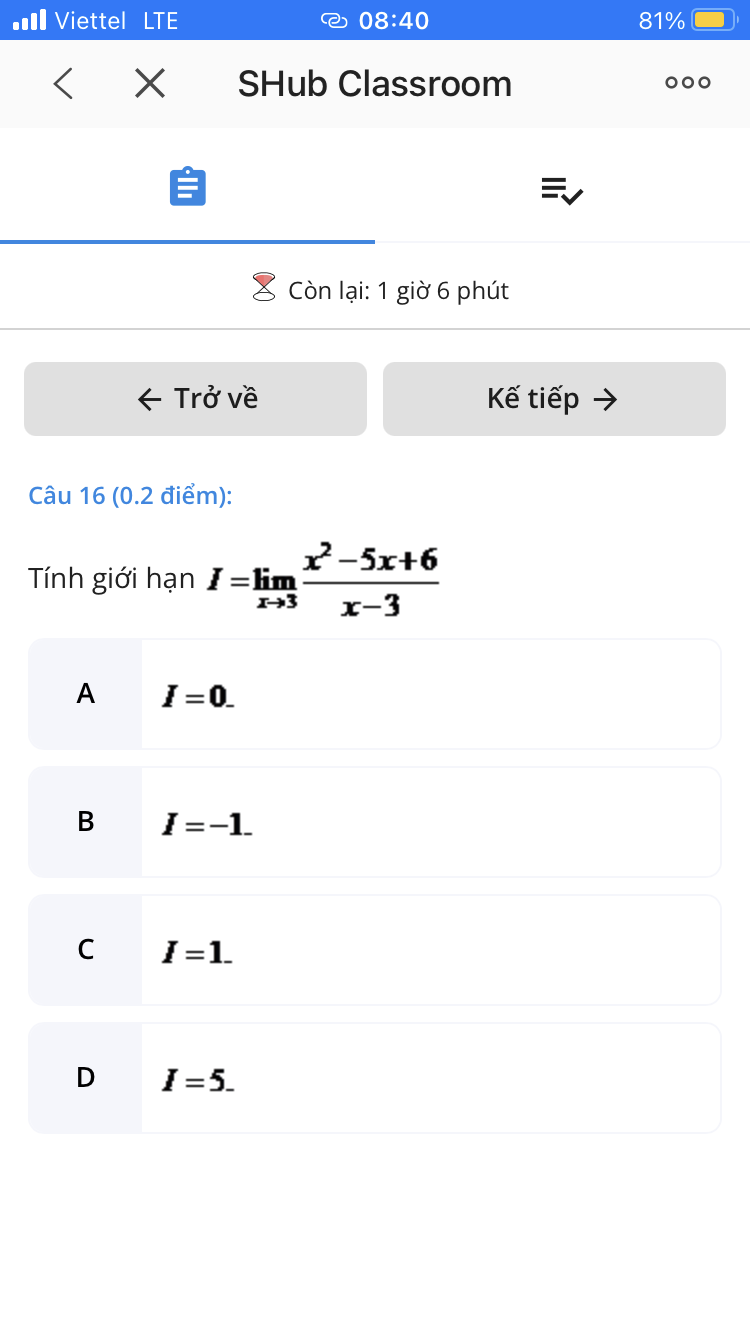
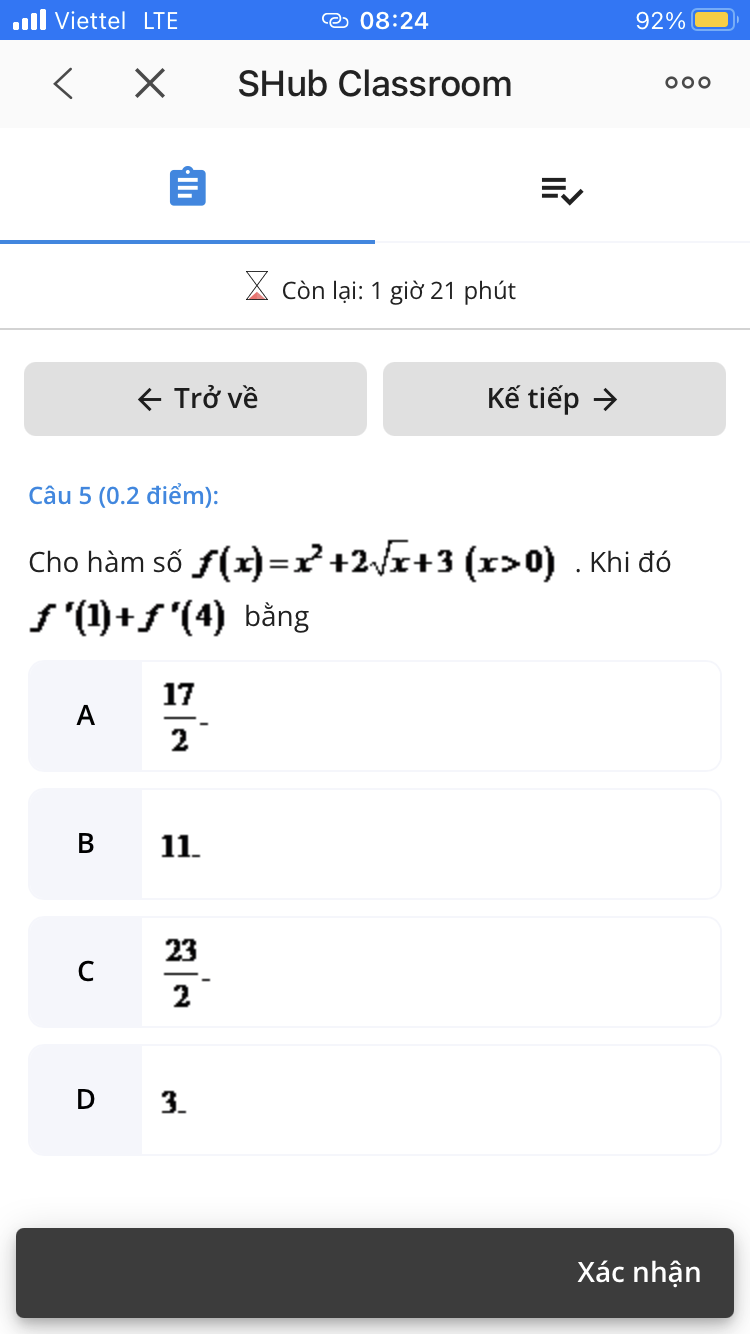
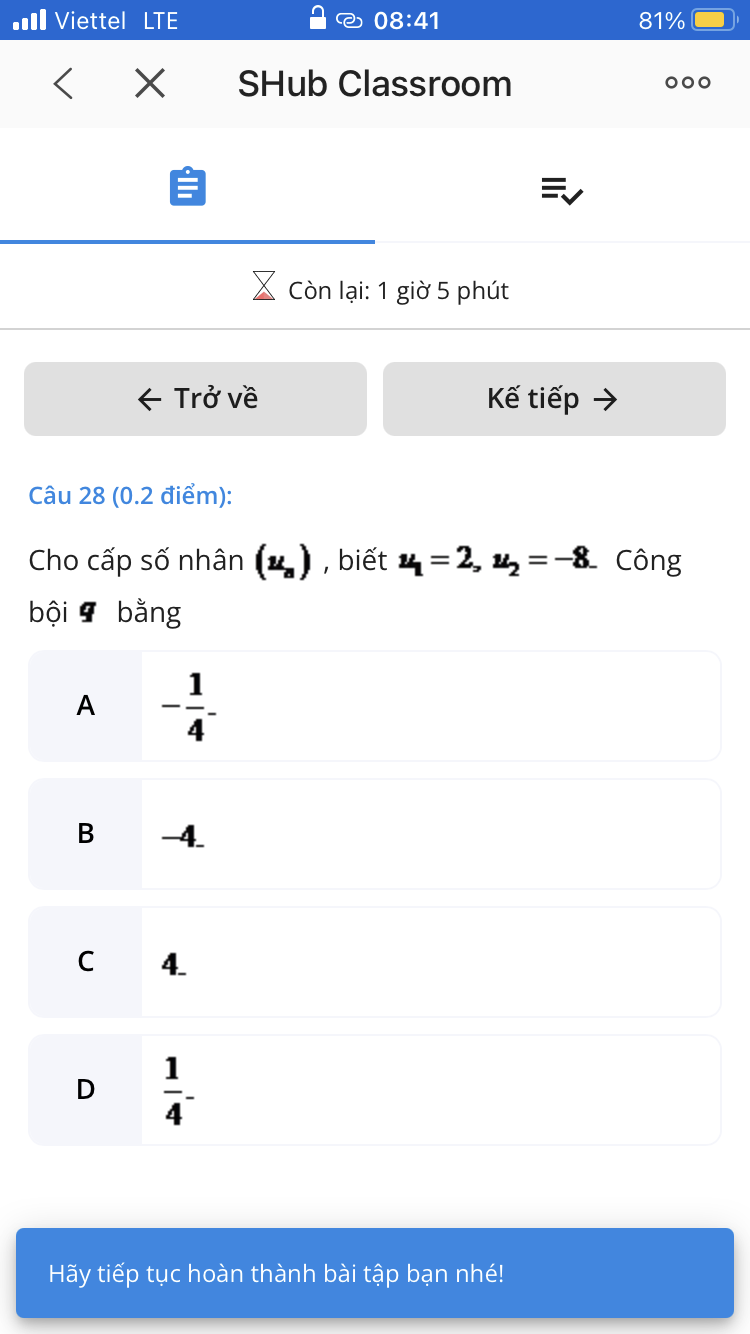
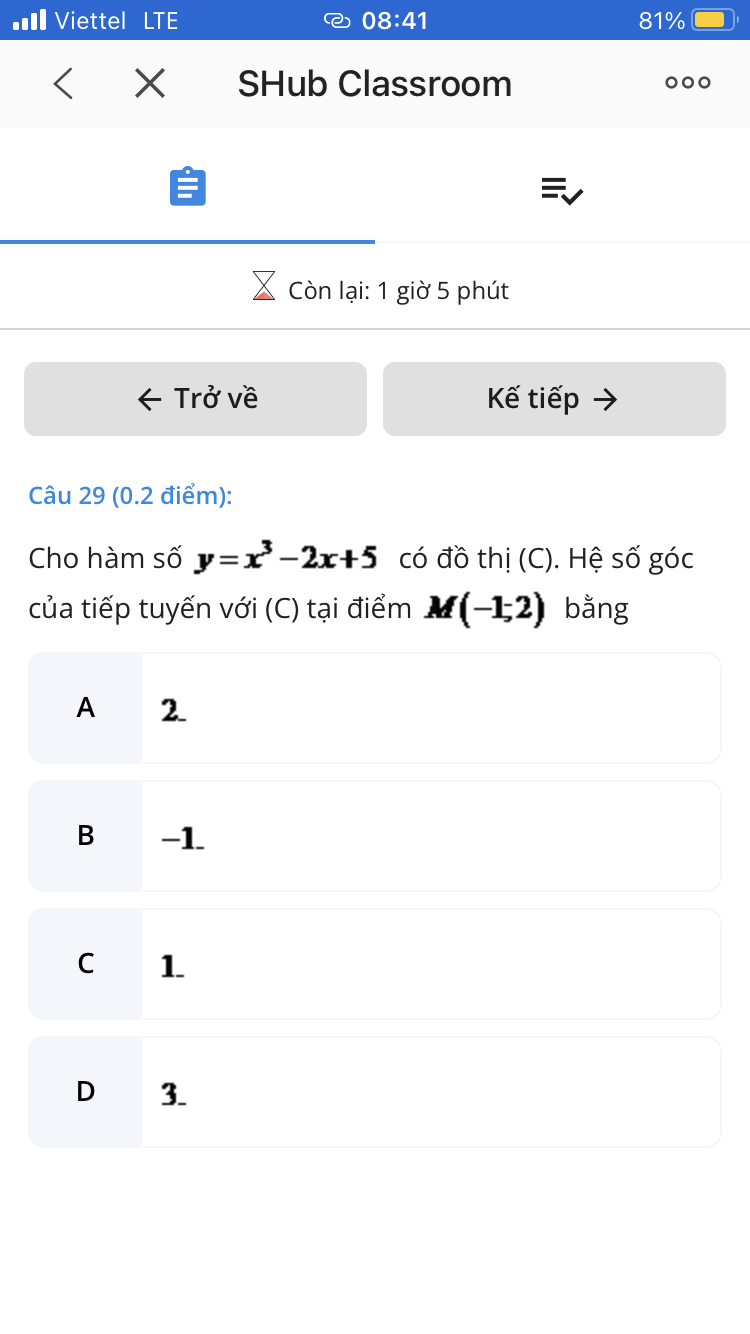
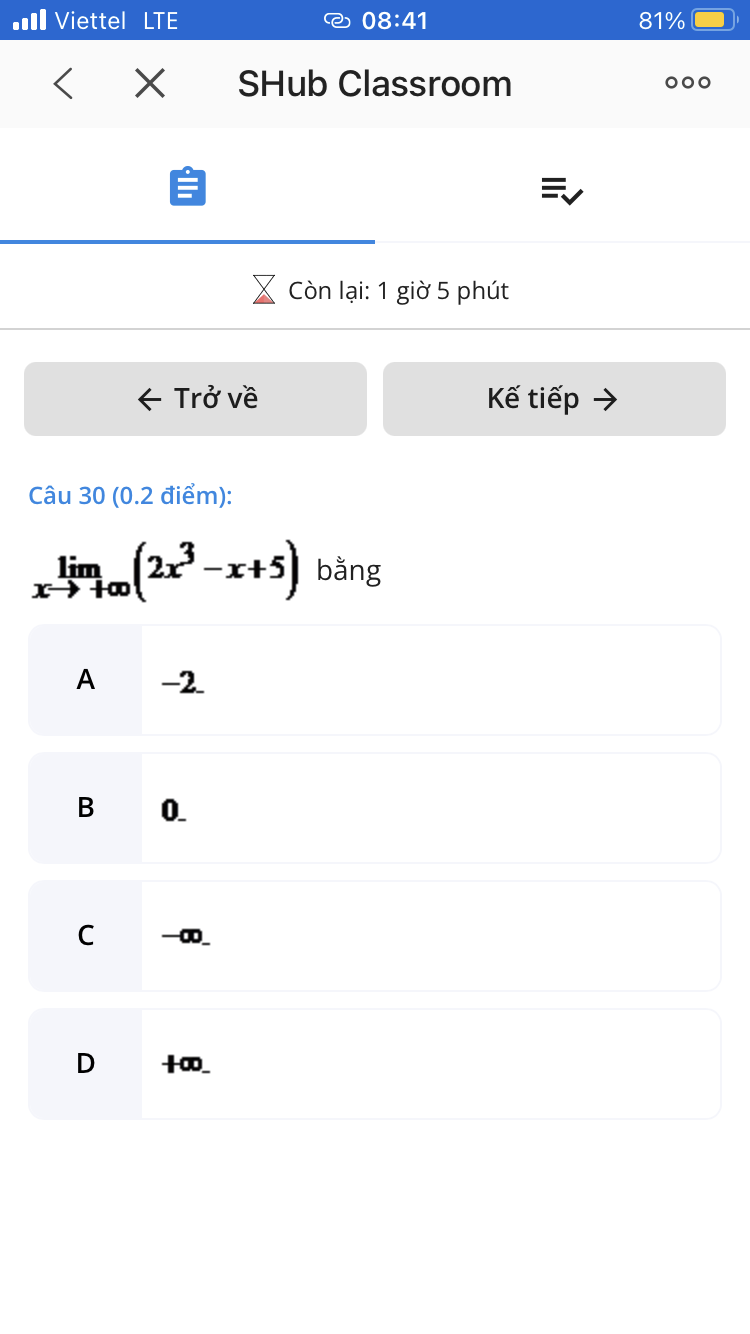
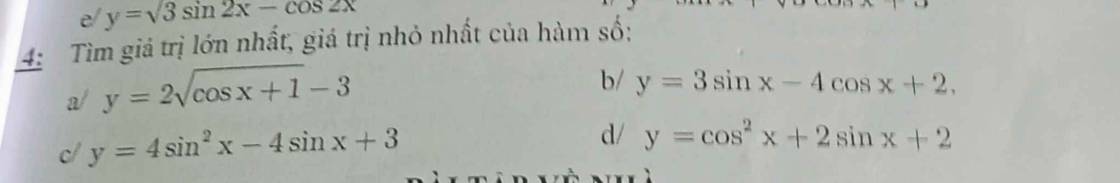a: \(\lim\limits_{x\rightarrow+\infty}\left(x^2+3\right)=\lim\limits_{x\rightarrow+\infty}x^2+\lim\limits_{x\rightarrow+\infty}3=+\infty\)
b: \(\lim\limits_{x\rightarrow-\infty}\left(\sqrt{x^2+x}-x\right)\)
\(=\lim\limits_{x\rightarrow-\infty}\dfrac{x^2+x-x^2}{\sqrt{x^2+x}+x}=\lim\limits_{x\rightarrow-\infty}\dfrac{x}{\sqrt{x^2+x}+x}\)
\(=\lim\limits_{x\rightarrow-\infty}\dfrac{1}{-\sqrt{1+\dfrac{1}{x}}+1}=-\infty\)
c: \(\lim\limits_{x\rightarrow-\infty}\dfrac{1}{x+2}=\lim\limits_{x\rightarrow-\infty}\dfrac{\dfrac{1}{x}}{1+\dfrac{2}{x}}=0\)
d: \(\lim\limits_{x\rightarrow+\infty}\sqrt{\dfrac{2x}{x+3}}=\lim\limits_{x\rightarrow+\infty}\sqrt{\dfrac{2}{1+\dfrac{3}{x}}}=\sqrt{\dfrac{2}{1}}=\sqrt{2}\)