a) Ta có:
Q(0) = -2.0⁴ + 4.0³ - 3.0² + 0
= 0
⇒ x = 0 là một nghiệm của Q(x)
P(0) = 6.0² - 5.0 - 1
= 0 - 0 - 1
= -1
Vậy x = 0 không là nghiệm của P(x)
b) Cho P(x) = 0
6x² - 5x - 1 = 0
6x² - 6x + x - 1 = 0
(6x² - 6x) + (x - 1) = 0
6x(x - 1) + (x - 1) = 0
(x - 1)(6x + 1) = 0
⇒ x - 1 = 0 hoặc 6x + 1 = 0
*) x - 1 = 0
x = 1
*) 6x + 1 = 0
6x = -1
x = -1/6
Vậy nghiệm của đa thức P(x) là: x = -1/6; x = 1
a,
\(P\left(x\right)=6x^2-5x-1=0\\ \Leftrightarrow6x^2-6x+x-1=0\\ \Leftrightarrow6x\left(x-1\right)+\left(x-1\right)=0\\ \Leftrightarrow\left(6x+1\right)\left(x-1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}6x+1=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{1}{6}\\x=1\end{matrix}\right.\)
Vậy x=0 không phải nghiệm của đa thức P(x). Nghiệm của đa thức P(x): x=-1/6 hoặc x=1
\(Q\left(x\right)=-2x^4+4x^3-3x^2+x=0\\ \Leftrightarrow x\left(-2x^3+4x^2-3x+1\right)=0\\ \Leftrightarrow x\left(-2x^3+2x^2+2x^2-2x-x+1\right)=0\\ \Leftrightarrow x\left[2x^2\left(1-x\right)-2x\left(1-x\right)+\left(1-x\right)\right]=0\\ \Leftrightarrow x\left[\left(2x^2-2x+1\right)\left(1-x\right)\right]=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\1-x=0\\2x^2-2x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\\2\left(x^2-x+\dfrac{1}{4}\right)=-\dfrac{1}{2}\left(vô.lí\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=1\end{matrix}\right.\)
Vậy x=0 là nghiệm của đa thức Q(x)
giúp mình với mn ơi mình cần giúp khẩn cấp T_T
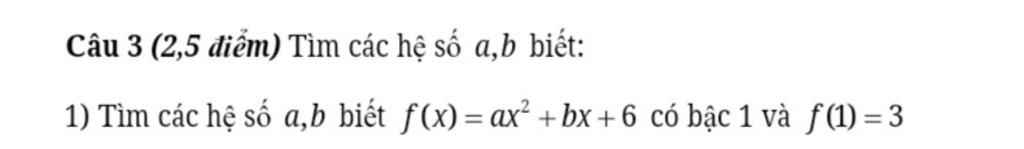
giúp mình với ạ,mình xin cảm ơn trước