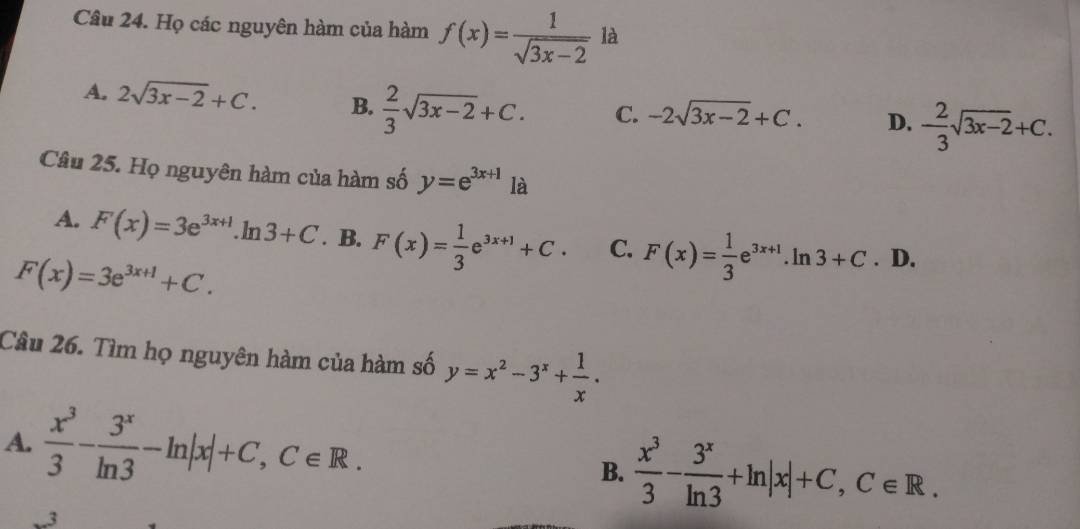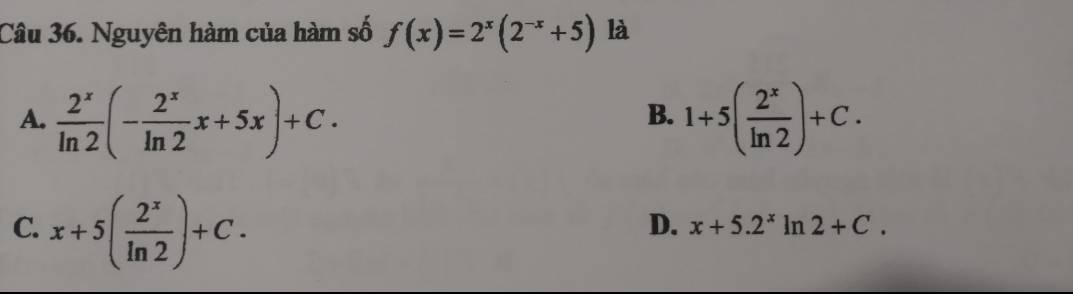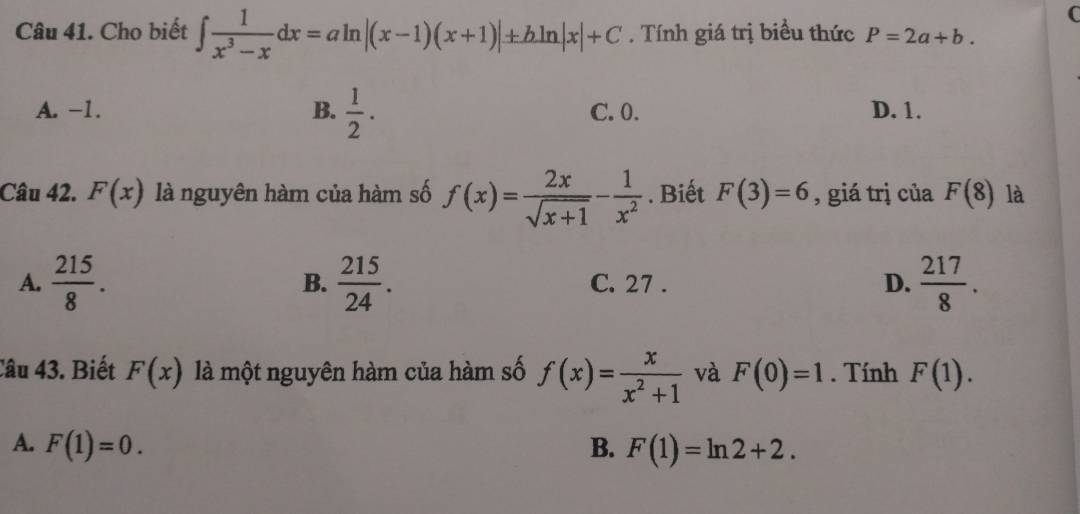42.
ĐKXĐ: \(x>0\)
\(log_3^2x-5log_3x+6\le0\)
\(\Leftrightarrow\left(log_3x-2\right)\left(log_3x-3\right)\le0\)
\(\Rightarrow2\le log_3x\le3\)
\(\Rightarrow9\le x\le27\)
\(\Rightarrow2a-b=9.2-27=\)
43.
\(r=\dfrac{1}{2};h=1\)
\(\Rightarrow V=\pi r^2h=\dfrac{\pi}{4}\)
44.
ĐKXĐ: \(a>0\)
\(log_2a+log_23=log_2\left(2a+2\right)\)
\(\Leftrightarrow log_2\left(3a\right)=log_2\left(2a+2\right)\)
\(\Rightarrow3a=2a+2\)
\(\Rightarrow a=2\)
45.
\(V=\dfrac{1}{3}.6a.20a^2=40a^3\)
46.
Pt hoành độ giao điểm:
\(-x^2+2=x^3+2\Leftrightarrow x^3+x^2=0\)
\(\Leftrightarrow x^2\left(x+1\right)=0\Rightarrow\left[{}\begin{matrix}x=0\\x=-1\end{matrix}\right.\)
\(\Rightarrow\) Hai đồ thị có 2 giao điểm
47.
\(y'=x^2-5x+6=0\Rightarrow\left[{}\begin{matrix}x=2\\x=3\end{matrix}\right.\)
\(f\left(1\right)=\dfrac{29}{6}\) ; \(f\left(2\right)=\dfrac{17}{3}\) ; \(f\left(3\right)=\dfrac{11}{2}\)
\(\Rightarrow\) Hàm đạt min tại \(x=1\) và đạt max tại \(x=2\)
\(\Rightarrow x_1+x_2=3\)
48.
\(y'=-4x^3=0\Rightarrow x=0\)
Do \(a=-1< 0\Rightarrow\)hàm đồng biến trên \(\left(-\infty;0\right)\)
49.
\(y'=3ax^2+2bx+c\Rightarrow\left\{{}\begin{matrix}c=0\\12a+4b+c=0\end{matrix}\right.\)
\(x=0;y=d\Rightarrow d=2\)
\(x=2;y=-2\Rightarrow8a+4b+2c+d=-2\)
\(\Rightarrow8a+4b+2=-2\Rightarrow\left\{{}\begin{matrix}12a+4b=0\\8a+4b=-4\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}a=1\\b=-3\end{matrix}\right.\)
\(\Rightarrow y=x^3-3x^2+2\)
\(\Rightarrow y\left(-2\right)=-18\)