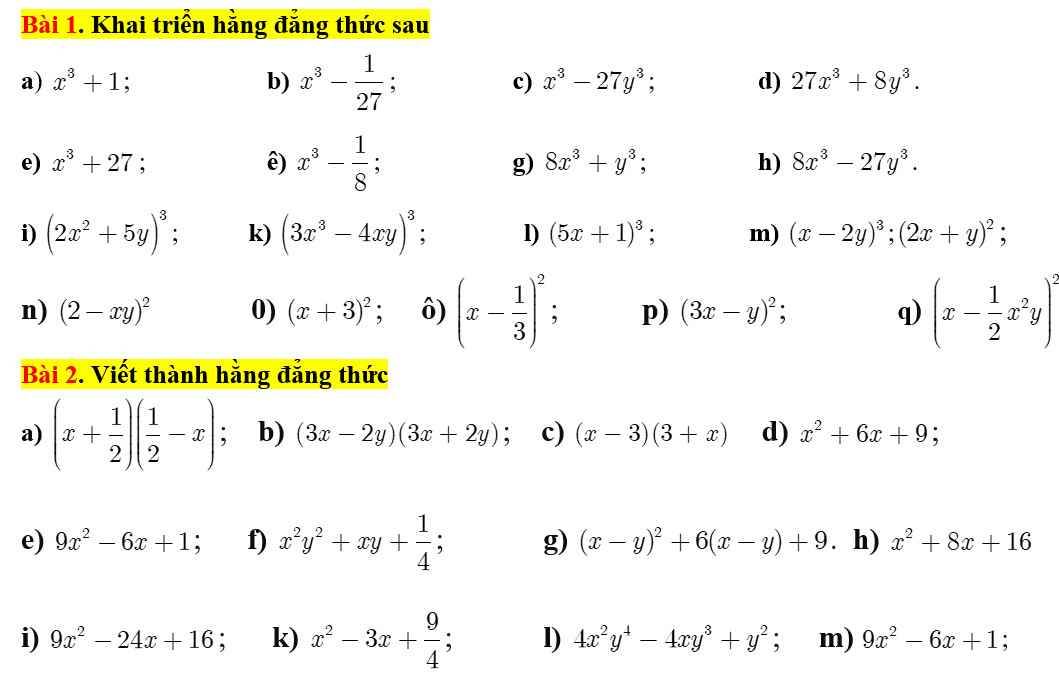
B2: a) \(\left(x+\dfrac{1}{2}\right)\left(\dfrac{1}{2}-x\right)\)
\(=-\left(x+\dfrac{1}{2}\right)\left(x-\dfrac{1}{2}\right)\)
\(=-x^2+\dfrac{1}{4}\)
b) \(\left(3x-2y\right)\left(3x+2y\right)\)
\(=\left(3x\right)^2-\left(2y\right)^2\)
\(=9x^2-4y^2\)
c) \(\left(x-3\right)\left(3+x\right)\)
\(=x^2-3^2\)
\(=x^2-9\)
d) \(x^2+6x+9\)
\(=x^2+2\cdot3\cdot x+3^2\)
\(=\left(x+3\right)^2\)
e) \(9x^2-6x+1\)
\(=\left(3x\right)^2-2\cdot3x\cdot1+1^2\)
\(=\left(3x-1\right)^2\)
f) \(x^2y^2+xy+\dfrac{1}{4}\)
\(=\left(xy\right)^2+2\cdot\dfrac{1}{2}\cdot xy+\left(\dfrac{1}{2}\right)^2\)
\(=\left(xy+\dfrac{1}{2}\right)^2\)
g) \(\left(x-y\right)^2+6\left(x-y\right)+9\)
\(=\left(x-y\right)^2+2\cdot3\cdot\left(x-y\right)+3^2\)
\(=\left(x-y+3\right)^2\)
h) \(x^2+8x+16\)
\(=x^2+2\cdot4\cdot x+4^2\)
\(=\left(x+4\right)^2\)
i) \(9x^2-24x+16\)
\(=\left(3x\right)^2-2\cdot3x\cdot4+4^2\)
\(=\left(3x-4\right)^2\)
k) \(x^2-3x+\dfrac{9}{4}\)
\(=x^2-2\cdot\dfrac{3}{2}\cdot x+\left(\dfrac{3}{2}\right)^2\)
\(=\left(x-\dfrac{3}{2}\right)^2\)
l) \(4x^2y^4-4xy^3+y^2\)
\(=\left(2xy^2\right)^2-2\cdot2xy^2\cdot y+y^2\)
\(=\left(2xy^2-y\right)^2\)
m) \(9x^2-6x+1\)
\(=\left(3x\right)^2-2\cdot3x\cdot1+1\)
\(=\left(3x-1\right)^2\)