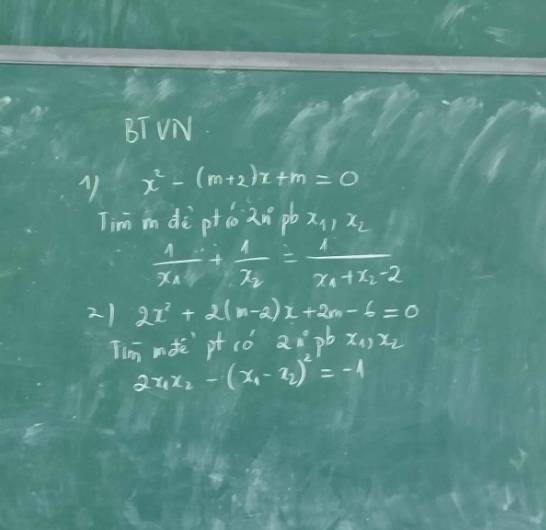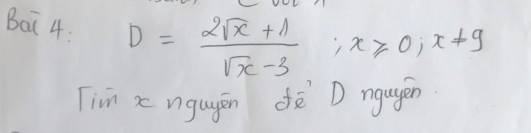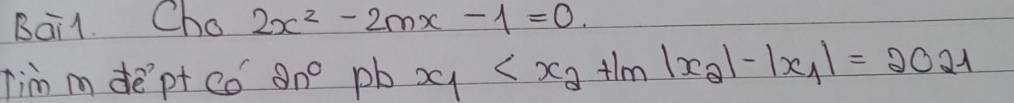1.
\(\Delta=\left(m+2\right)^2-4m=m^2+4>0;\forall m\)
\(\Rightarrow\) Phương trình luôn có 2 nghiệm pb với mọi m
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=m+2\\x_1x_2=m\end{matrix}\right.\)
\(\dfrac{1}{x_1}+\dfrac{1}{x_2}=\dfrac{1}{x_1+x_2-2}\)
\(\Leftrightarrow\dfrac{x_1+x_2}{x_1x_2}=\dfrac{1}{x_1+x_2-2}\)
\(\Leftrightarrow\dfrac{m+2}{m}=\dfrac{1}{m+2-2}\)
\(\Leftrightarrow\dfrac{m+2}{m}=\dfrac{1}{m}\)
\(\Rightarrow\left\{{}\begin{matrix}m\ne0\\m+2=1\end{matrix}\right.\) \(\Rightarrow m=-1\) (thỏa)
2.
\(\Delta'=\left(m-2\right)^2-2\left(2m-6\right)=\left(m-4\right)^2\)
Pt có 2 nghiệm pb khi \(\left(m-4\right)^2>0\Rightarrow m\ne4\)
Khi đó theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=-m+2\\x_1x_2=m-3\end{matrix}\right.\)
\(2x_1x_2-\left(x_1-x_2\right)^2=-1\)
\(\Leftrightarrow2x_1x_2-\left(x_1+x_2\right)^2+4x_1x_2=-1\)
\(\Leftrightarrow6\left(m-3\right)-\left(-m+2\right)^2=-1\)
\(\Leftrightarrow-m^2+10m-21=0\Rightarrow\left[{}\begin{matrix}m=3\\m=7\end{matrix}\right.\) (thỏa mãn)