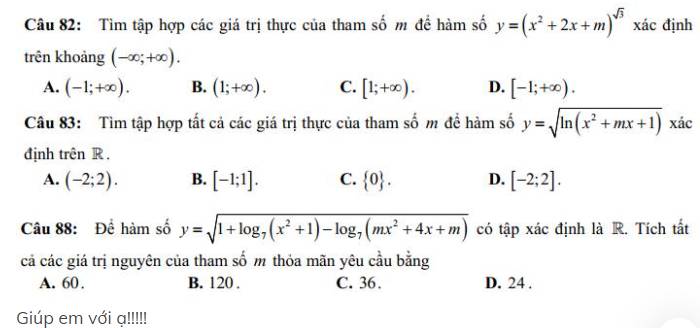
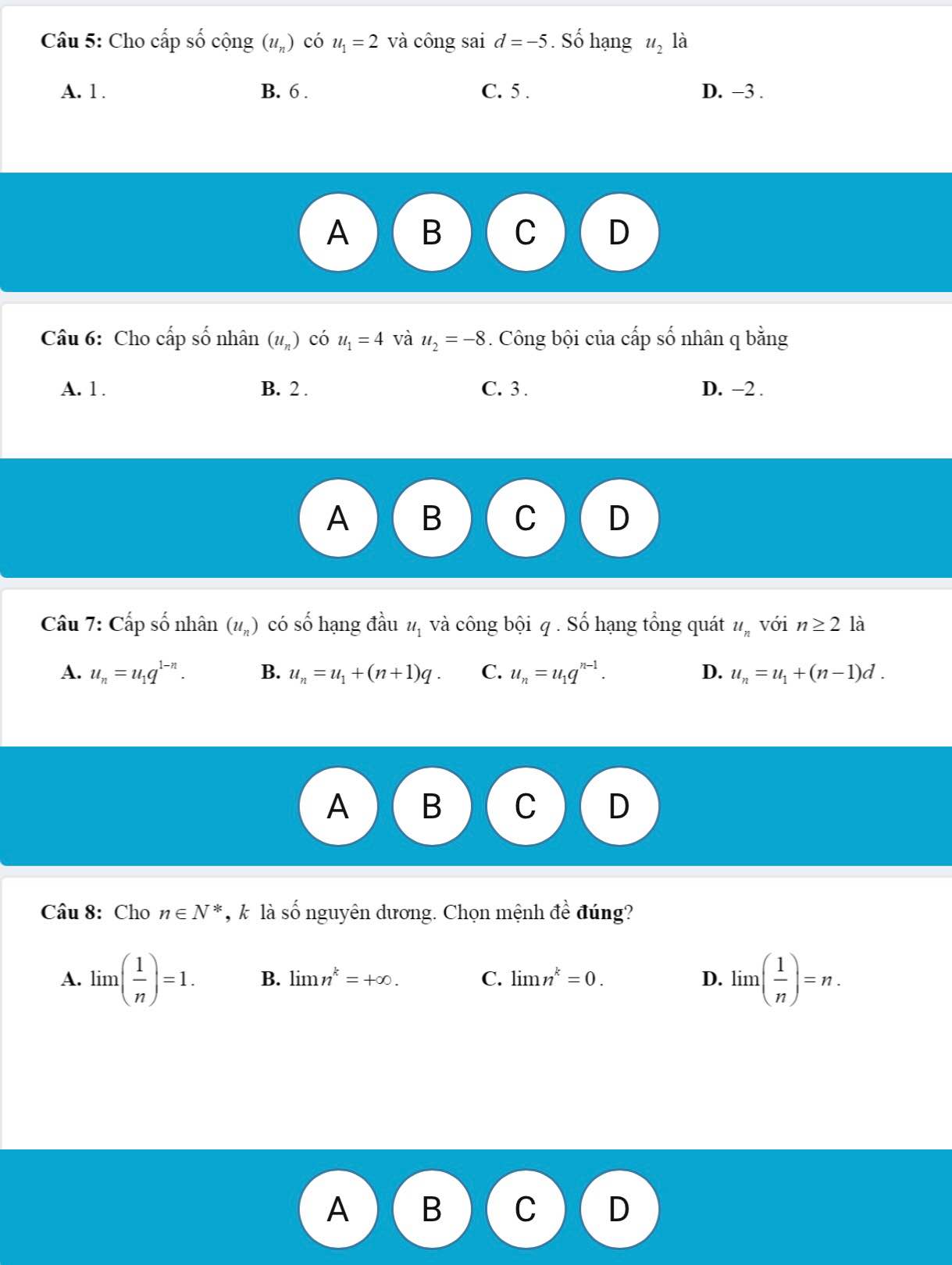
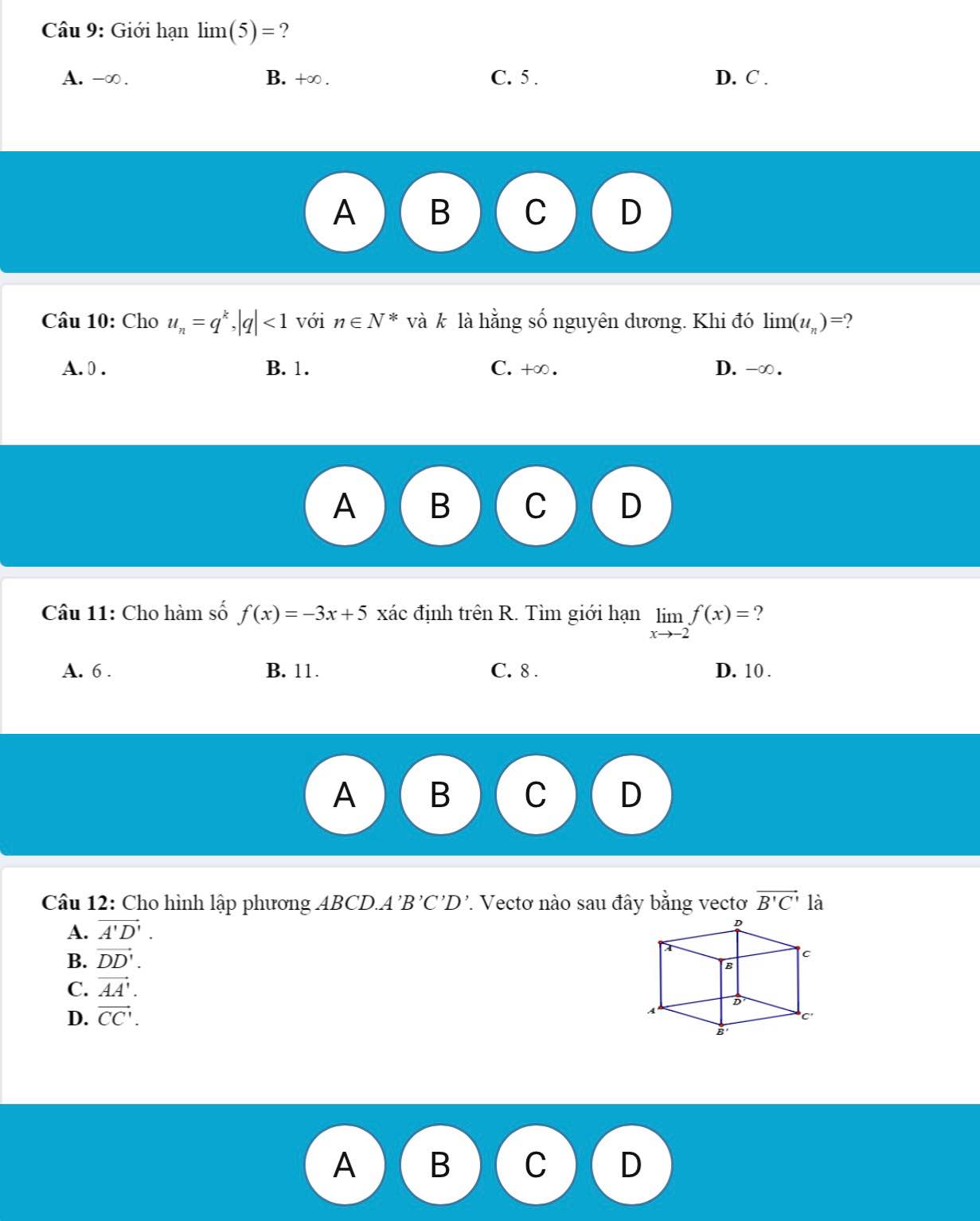
83.
\(\Leftrightarrow ln\left(x^2+mx+1\right)\ge0;\forall x\)
\(\Leftrightarrow x^2+mx+1\ge1;\forall x\)
\(\Leftrightarrow x^2+mx\ge0;\forall x\)
\(\Leftrightarrow\left\{{}\begin{matrix}a=1>0\\\Delta=m^2\le0\end{matrix}\right.\)
\(\Leftrightarrow m^2\le0\)
\(\Rightarrow m=0\)
C đúng
88.
\(\Leftrightarrow\left\{{}\begin{matrix}mx^2+4x+m>0\left(1\right)\\1+log_7\left(x^2+1\right)-log_7\left(mx^2+4x+m\right)\ge0\left(2\right)\end{matrix}\right.\)
Xét (1)
- Với \(m=0\) ko thỏa mãn
- Với \(m\ne0\Rightarrow\left\{{}\begin{matrix}m>0\\\Delta'=4-m^2< 0\\\end{matrix}\right.\) \(\Rightarrow m>2\)
Khi \(mx^2+4x+m>0\), xét (2):
\(\Leftrightarrow log_77\left(x^2+1\right)-log_7\left(mx^2+4x+m\right)\ge0\)
\(\Leftrightarrow log_7\dfrac{7\left(x^2+1\right)}{mx^2+4x+m}\ge0\)
\(\Leftrightarrow\dfrac{7\left(x^2+1\right)}{mx^2+4x+m}\ge1\)
\(\Leftrightarrow7x^2+7\ge mx^2+4x+m\)
\(\Leftrightarrow\left(m-7\right)x^2+4x+m-7\le0\)
\(\Leftrightarrow\left\{{}\begin{matrix}m-7< 0\\\Delta'=4-\left(m-7\right)^2\le0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< 7\\\left(9-m\right)\left(m-5\right)\le0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< 7\\\left[{}\begin{matrix}m\ge9\\m\le5\end{matrix}\right.\end{matrix}\right.\) \(\Rightarrow m\le5\)
\(\Rightarrow2< m\le5\Rightarrow m=\left\{3;4;5\right\}\)
A đúng