a.
\(\Leftrightarrow2cos^2x-1-\sqrt{3}sin2x-\sqrt{3}sinx-cosx+4=0\)
\(\Leftrightarrow2cos^2x-2\sqrt{3}sinx.cosx-\sqrt{3}sinx-cosx+3=0\)
\(\Leftrightarrow4cos^2x-4\sqrt{3}sinx.cosx-2\sqrt{3}sinx-2cosx+6=0\)
\(\Leftrightarrow4cos^2x-4\sqrt{3}sinx.cosx-2\sqrt{3}sinx-2cosx+2+4\left(sin^2x+cos^2x\right)=0\)
\(\Leftrightarrow\dfrac{1}{2}\left(4cos^2x-4cosx+1\right)+\dfrac{1}{2}\left(4sin^2x-4\sqrt{3}sinx+3\right)+2\left(3cos^2x-2\sqrt{3}sinx.cosx+sin^2x\right)=0\)
\(\Leftrightarrow\dfrac{1}{2}\left(2cosx-1\right)^2+\dfrac{1}{2}\left(2sinx-\sqrt{3}\right)^2+2\left(\sqrt{3}cosx-sinx\right)^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}2cosx-1=0\\2sinx-\sqrt{3}=0\\\sqrt{3}cosx-sinx=0\end{matrix}\right.\)
\(\Leftrightarrow x=\dfrac{\pi}{3}+k2\pi\)
b.
\(\Leftrightarrow\left(3tan^2x-2\sqrt{3}tanx+1\right)+\left(4sin^2x-4sinx+1\right)=0\)
\(\Leftrightarrow\left(\sqrt{3}tanx-1\right)^2+\left(2sinx-1\right)^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}\sqrt{3}tanx-1=0\\2sinx-1=0\end{matrix}\right.\)
\(\Leftrightarrow x=\dfrac{\pi}{6}+k2\pi\)
c.
Ta có:
\(VP=2\left(1+sin^22x\right)\ge2\left(1+0\right)=2\)
\(VT=cos3x+\sqrt{2-cos^23x}\le\sqrt{2\left(cos^23x+2-cos^23x\right)}=2\)
\(\Rightarrow VT\le VP\)
Đẳng thức xảy ra khi và chỉ khi:
\(\left\{{}\begin{matrix}sin2x=0\\cos3x=\sqrt{2-cos^23x}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sin2x=0\\cos3x=1\end{matrix}\right.\)
\(\Leftrightarrow x=k2\pi\)
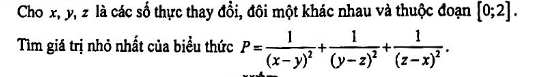
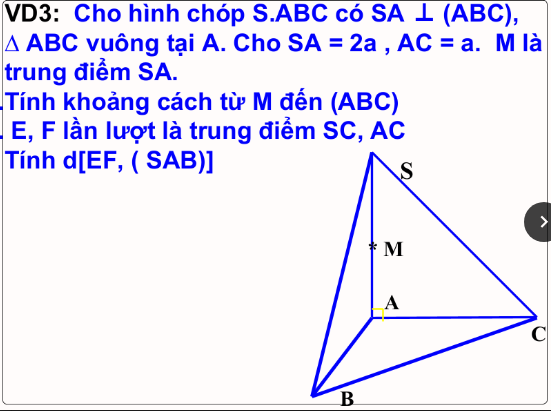
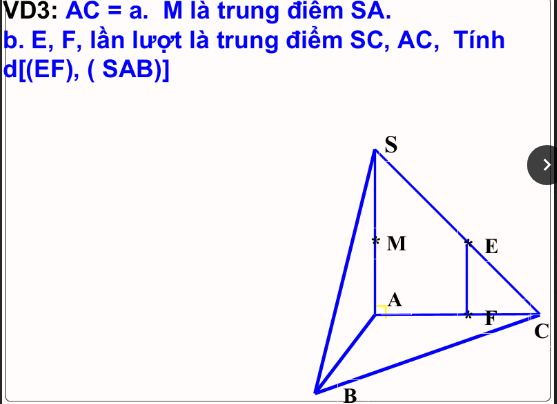
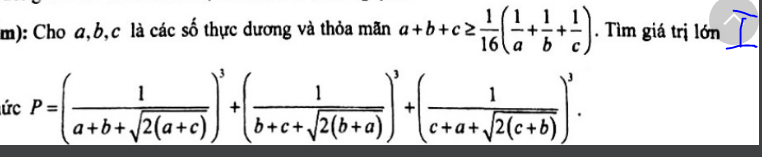mọi người giúp em với em cảm ơn nhiều lắmmmmm
