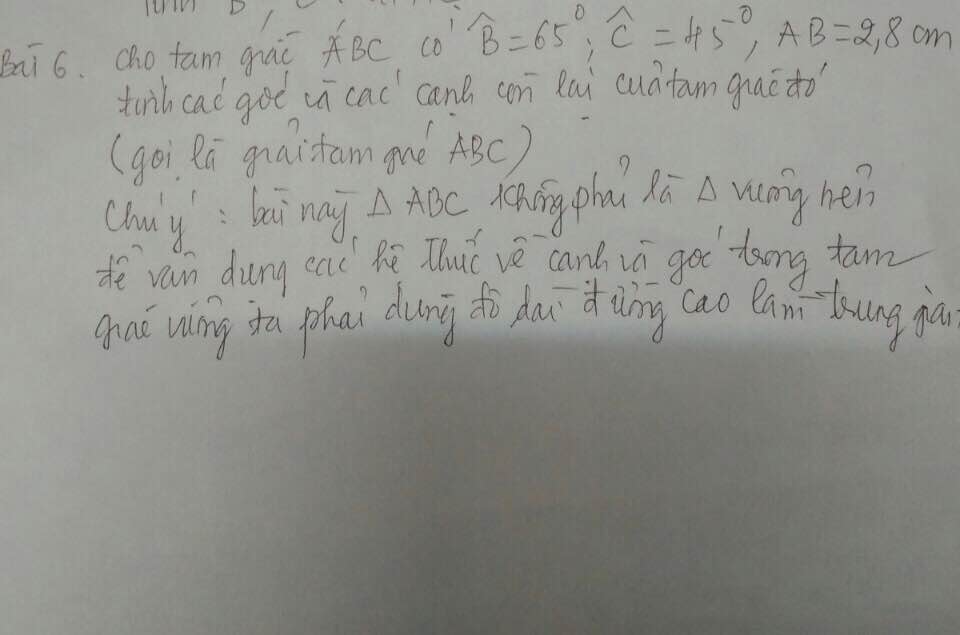\(Pt\Leftrightarrow9x^2+25y^2+576-96xy-144x+768y=9x^2+16x+32\)
\(\Leftrightarrow25y^2+96\cdot\left(8-x\right)y+544-160x=0\)
Phương trình có nghiệm nguyên khi
\(\left\{{}\begin{matrix}\Delta_y'=48^2\left(8-x\right)^2-25\left(544-160x\right)>0\left(1\right)\\S=-\dfrac{96}{25}\left(8-x\right)\in Z\left(2\right)\\P=\dfrac{544-160x}{25}\in Z\left(3\right)\end{matrix}\right.\)
\(\left(1\right)\Leftrightarrow2304x^2-32864x+133856>0\)
\(\Leftrightarrow x^2-14,26x+58,14>0,\forall x\in R\left(\Delta=-29,14< 0;a=1>0\right)\)
\(\left(2\right)\Leftrightarrow8-x⋮25\)
\(\Leftrightarrow x\equiv8\left(mod25\right)\left(4\right)\)
\(\left(3\right)\Leftrightarrow544-160x⋮25\)
\(\Leftrightarrow544-160x\equiv0\left(mod25\right)\)
mà \(544\equiv19\left(mod25\right);160\equiv10\left(mod25\right)\)
\(\Rightarrow19-10x\equiv0\left(mod25\right)\)
\(\Rightarrow10x\equiv19\left(mod25\right)\)
\(\Rightarrow x=19.15\left(mod25\right)\left(vì.15.10=150\right)\)
\(\Rightarrow x\equiv10\left(mod25\right)\left(5\right)\left(vì.19.25=285\Rightarrow285\left(mod25\right)=10\right)\)
\(\left(4\right);\left(5\right)\) ta thấy mâu thuẫn nên \(x\in\varnothing\)
Vậy phương trình cho không tồn tại cặp \(\left(x;y\right)\in Z\)