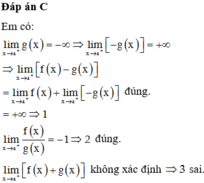Các câu hỏi tương tự
cho \(f\left(x\right)=\left\{{}\begin{matrix}x^2-3\\x+3\end{matrix}\right.\) \(x\ge3\);\(x< 3\)
a) tính \(\lim\limits_{x\rightarrow3^+}f\left(x\right)=?\)
\(\lim\limits_{x\rightarrow3^-}f\left(x\right)=?\)
b) tính \(\lim\limits_{x\rightarrow3}f\left(x\right)\) nếu có
Cho f(x) là hàm đa thức thỏa \(\lim\limits_{x\rightarrow2}\dfrac{f\left(x\right)+1}{x-2}=a\left(a\in R\right)\) và tồn tại \(\lim\limits_{x\rightarrow2}\dfrac{\sqrt{f\left(x\right)+2x+1}-x}{x^2-4}=T\left(T\in R\right).\) Tìm T theo a.
a) limlimits_{xrightarrow+infty}^{3_{sqrt{x^3+4x^2}-x}}b) fleft(xright)left{{}begin{matrix}dfrac{4x-1}{x-1}neux17x+1neux 1end{matrix}right. Tính limlimits fleft(xright)_{xrightarrow1^+} , limlimits fleft(xright)_{xrightarrow1^-}
Đọc tiếp
a) \(\lim\limits_{x\rightarrow+\infty}\)\(^{3_{\sqrt{x^3+4x^2}-x}}\)
b) \(f\left(x\right)=\left\{{}\begin{matrix}\dfrac{4x-1}{x-1}neux>1\\7x+1neux< 1\end{matrix}\right.\)
Tính \(\lim\limits f\left(x\right)_{x\rightarrow1^+}\) , \(\lim\limits f\left(x\right)_{x\rightarrow1^-}\)
e) lim\(\dfrac{17}{x^2+1}\)(x-->+\(\infty\))
f) lim\(\dfrac{-2x^2+x-1}{3+x}\)(x-->+\(\infty\))
#HELP
Hình bên là đồ thị của một hàm số y=f(x) y=f(x) , f(x) f(x) không xác định tại x = -1 x=−1 . Dự đoán \lim\limits_{x\rightarrow-1}f\left(x\right) x→−1 lim f(x) .
#THANKS _YOU
cho hàm số yfleft(xright) liên tục trên R thỏa limlimits_{xrightarrow-infty}fleft(xright)+infty , limlimits_{xrightarrow+infty}fleft(xright)-dfrac{1}{2}tìm số đường tiệm cận củ đồ thị hàm số đã cholimlimits_{xrightarrow-infty}fleft(xright)+infty
Đọc tiếp
cho hàm số \(y=f\left(x\right)\) liên tục trên R thỏa
\(\lim\limits_{x\rightarrow-\infty}f\left(x\right)=+\infty\) , \(\lim\limits_{x\rightarrow+\infty}f\left(x\right)=-\dfrac{1}{2}\)
tìm số đường tiệm cận củ đồ thị hàm số đã cho
\(\lim\limits_{x\rightarrow-\infty}f\left(x\right)=+\infty\)
Cho
f
(
x
)
x
2018
1009
x
2
+
2019
x
Giá trị của
lim
∆
x
→
0
f
(
∆
x
+
1
)...
Đọc tiếp
Cho f ( x ) = x 2018 = 1009 x 2 + 2019 x Giá trị của lim ∆ x → 0 f ( ∆ x + 1 ) - f ( 1 ) ∆ x bằng
A. 1009
B. 1008
C. 2018
D. 2019
Cho hàm số
y
f
x
a
x
5
+
b
x
3
+
c
x
+
d
a
,
b
,
c
,
d
∈
ℝ
;
a
≠...
Đọc tiếp
Cho hàm số y = f x = a x 5 + b x 3 + c x + d a , b , c , d ∈ ℝ ; a ≠ 0 . Biết f'(-1)=3 . Tính lim ∆ x → 0 f 1 + ∆ x - f 1 ∆ x
A. 3
B. -3
C. 1
D. -1
Tính các giới hạn sau:
a) $\underset{x\to 3}{\mathop{\lim }}\,\left( x+2 \right);$
b) $\underset{x\to +\infty }{\mathop{\lim }}\,\left( {{x}^{2}}-x+1 \right).$