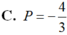\(\left\{{}\begin{matrix}3x-y\ge1\\2x+y\le6\\x+3y>3\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y\le3x-1\left(1\right)\\y\le6-2x\left(2\right)\\y>\dfrac{3-x}{3}\left(3\right)\end{matrix}\right.\)
Để hệ bất phương trình có nghiệm, ta cần tìm các giá trị của \(\text{x}\) thỏa mãn đồng thời cả ba bất phương trình trên.
\(\left(1\right);\left(2\right)\Leftrightarrow3x+1\ge6-2x\)
\(\Leftrightarrow5x\ge5\)
\(\Leftrightarrow x\ge1\left(a\right)\)
\(\left(1\right);\left(3\right)\Leftrightarrow3x-1>\dfrac{3-x}{3}\)
\(\Leftrightarrow10x>6\)
\(\Leftrightarrow x>\dfrac{3}{5}\left(b\right)\)
\(\left(a\right);\left(b\right)\Leftrightarrow x\ge1\) thỏa mãn \(\left(3\right)\)
Vậy tập nghiệm của hệ bất phương trình là:
\(\left(x;y\right)=\left\{x\ge1;y>\dfrac{3-x}{3}\right\}\)