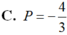\(\dfrac{f\left(x_1\right)-f\left(x_2\right)}{x_1-x_2}\)
\(=\dfrac{\left[x_1^2-2x_1\left(m+1\right)-3-x_2^2+2x_2\left(m-1\right)+3\right]}{x_1-x_2}\)
\(=x^1+x^2-2\left(m-1\right)\)
Vì \(x_1;x_2\in\left(4;2018\right)\)
nên \(\left\{{}\begin{matrix}x_1>4\\x_2>4\end{matrix}\right.\Leftrightarrow x_1+x_2>8\)
Để hàm số đồng biến trên khoảng (4;2018) thì \(8-2\left(m-1\right)>0\)
\(\Leftrightarrow2\left(m-1\right)< 8\)
hay m<5
Vậy: Chọn D
Đúng 0
Bình luận (0)
