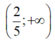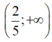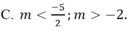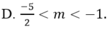\(\Leftrightarrow x^2-\left(2m-1\right)x+2m-2=0\) có 2 nghiệm pb \(x_1;x_2\) thỏa mãn \(\left|x_1-x_2\right|=5\)
\(\Delta=\left(2m-1\right)^2-4\left(2m-2\right)=4m^2-12m+9=\left(2m-3\right)^2\)
Pt có 2 nghiệm pb khi \(\left(2m-3\right)^2>0\Rightarrow m\ne\dfrac{3}{2}\)
Theo Viet: \(\left\{{}\begin{matrix}x_1+x_2=2m-1\\x_1x_2=2m-2\end{matrix}\right.\)
\(\left|x_1-x_2\right|=5\Leftrightarrow\left(x_1-x_2\right)^2=25\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-4x_1x_2=25\)
\(\Leftrightarrow\left(2m-1\right)^2-4\left(2m-2\right)=25\)
\(\Leftrightarrow\left(2m-3\right)^2=25\)
\(\Rightarrow\left[{}\begin{matrix}2m-3=5\\2m-3=-5\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}m=4\\m=-1\end{matrix}\right.\)