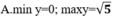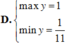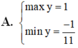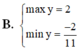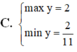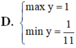\(-1< =sinx< =1\)
=>\(-2< =2\cdot sinx< =2\)
=>\(-2+3< =2\cdot sinx+3< =2+3\)
=>\(1< =2\cdot sinx+3< =5\)
=>\(\dfrac{2}{1}>=\dfrac{2}{2sinx+3}>=\dfrac{2}{5}\)
=>\(\dfrac{2}{5}< =y< =2\)
\(y_{min}=\dfrac{2}{5}\) khi sin x=1
=>\(x=\dfrac{\Omega}{2}+k2\Omega\)
\(y_{max}=2\) khi sin x=-1
=>\(x=-\dfrac{\Omega}{2}+k2\Omega\)
Tìm GTLN và GTNN của hàm sau: y = 2 sin x + 3
Tìm GTLN và GTNN của hàm số: y = 2 sin x + cos x + 3 2 cos x - sin x + 4
Tìm GTLN và GTNN của hàm số y = 2 sin x + cos x + 3 2 cos x - sin x + 4 là
Tìm GTLN,GTNN của hàm số:
a, \(y=3cosx-1\)
b, \(y=5+2sinx\)
c,\(y=\sqrt{3+cos2x}\)
d,\(y=\sqrt{5sinx-1}+2\)
Tìm GTLN và GTNN của hàm số y = 3 + sin2x
Tìm GTLN và GTNN của hàm số y = sin x + 2 cos x + 1 sin x + cos x + 3 (*)
Tìm GTLN và GTNN của hàm số sau: y = sin x + 2 cos x + 1 sin x + cos x + 2
A. min y = 0, max y = 1
B. min y= -2, max y= 1
C. min y =-1, max y= 1
D. Tất cả sai
Tìm GTLN/GTNN của hàm số: \(y=sin^4\left(x+\dfrac{\pi}{3}\right)+2\)
Tìm GTLN và GTNN của hàm số
y=3sinx + 4cosx + 5
A. min y = 0, max y= 13
B. min y =0, max y=10
C. min y= 1, max y=10
D. Tất cả sai
Tìm GTLN và GTNN của hàm số sau: y = 1 - 2 cos 2 x + 1
