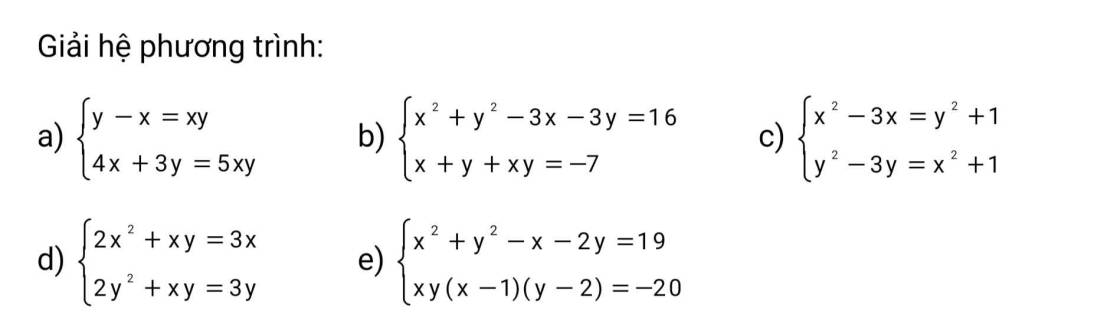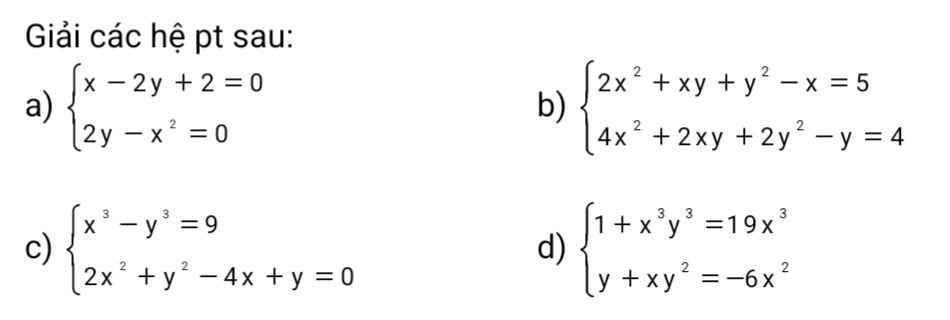Câu 4:
b: ĐKXĐ: \(x\in R\)
\(x+2+\left(x+1\right)\cdot\sqrt{2\left(x^2+4\right)}=x^2\)
=>\(\left(x+1\right)\cdot\sqrt{2\left(x^2+4\right)}-x^2+x+2=0\)
=>\(\left(x+1\right)\cdot\sqrt{2x^2+8}-\left(x^2-x-2\right)=0\)
=>\(\left(x+1\right)\cdot\sqrt{2x^2+8}-\left(x-2\right)\left(x+1\right)=0\)
=>\(\left(x+1\right)\left(\sqrt{2x^2+8}-\left(x-2\right)\right)=0\)
=>\(\left[{}\begin{matrix}x+1=0\\\sqrt{2x^2+8}=x-2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\left(nhận\right)\\\left\{{}\begin{matrix}x>=2\\2x^2+8=\left(x-2\right)^2\end{matrix}\right.\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=-1\\\left\{{}\begin{matrix}x>=2\\2x^2+8-x^2+4x-4=0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\\left\{{}\begin{matrix}x>=2\\x^2-4x+4=0\end{matrix}\right.\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=-1\\x=2\end{matrix}\right.\)
a: \(\left\{{}\begin{matrix}x+2y=5\\x-y=2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x+2y-x+y=5-2\\x-y=2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}3y=3\\x-y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=1\\x=2+y=2+1=3\end{matrix}\right.\)
Câu 3:
a:
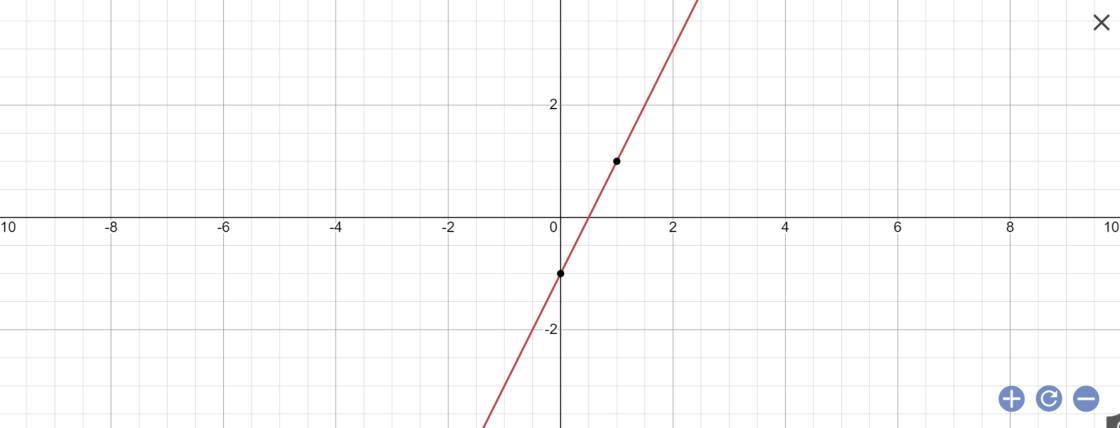
b: Để (d')//(d) thì \(\left\{{}\begin{matrix}m^2-2=2\\m-3\ne-1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}m^2=4\\m\ne2\end{matrix}\right.\Leftrightarrow m=-2\)