a) \(\dfrac{2\sqrt{x}}{\sqrt{x}+3}+\dfrac{\sqrt{x}}{\sqrt{x}-3}+\dfrac{3x+9}{9-x}\)
\(=\dfrac{2\sqrt{x}\left(\sqrt{x}-3\right)}{x-9}+\dfrac{\sqrt{x}\left(\sqrt{x}+3\right)}{x-9}-\dfrac{9+3x}{x-9}\)
\(=\dfrac{2x-6\sqrt{x}+x+3\sqrt{x}-9-3x}{x-9}\)
\(=\dfrac{-3\sqrt{x}-9}{x-9}\)
\(=\dfrac{-3\left(\sqrt{x}+3\right)}{x-9}\)
\(=\dfrac{-3}{\sqrt{x}-3}\)
b) Ta có: \(P>0\)
\(\Leftrightarrow\dfrac{-3}{\sqrt{x}-3}>0\)
\(\Leftrightarrow\sqrt{x}-3< 0\)(vì -3 < 0 nên muốn bt trên lớn hơn không thì ở dưới phải là số âm)
\(\Leftrightarrow\sqrt{x}< 3\)
\(\Leftrightarrow x< 9\)
So sánh với đkxđ ta được 0\(\le\)x\(< \)9
Chúc bạn học tốt <3
a: \(=\dfrac{2x-6\sqrt{x}+x+3\sqrt{x}-3x-9}{x-9}\)
\(=\dfrac{-3\sqrt{x}-3}{x-9}=-\dfrac{3}{\sqrt{x}-3}\)
b: Để P>0 thì căn x-3<0
=>0<=x<9

















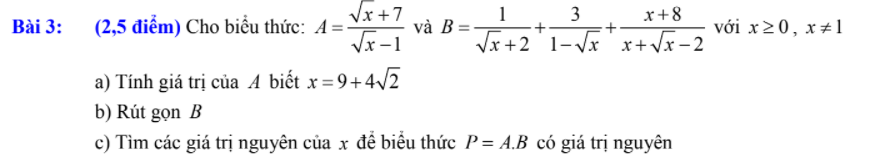 được không ạ, mai em phải nộp rồi, cảm ơn mn nhiều.
được không ạ, mai em phải nộp rồi, cảm ơn mn nhiều. 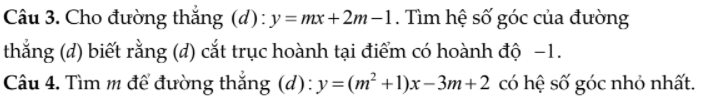 này được
này được