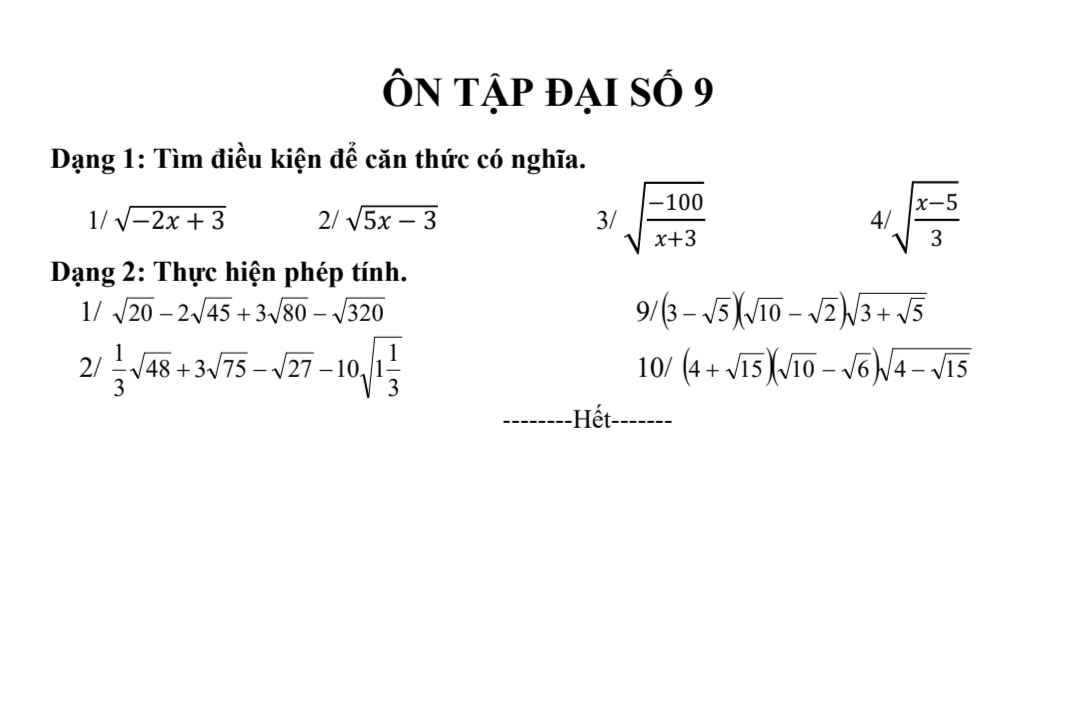
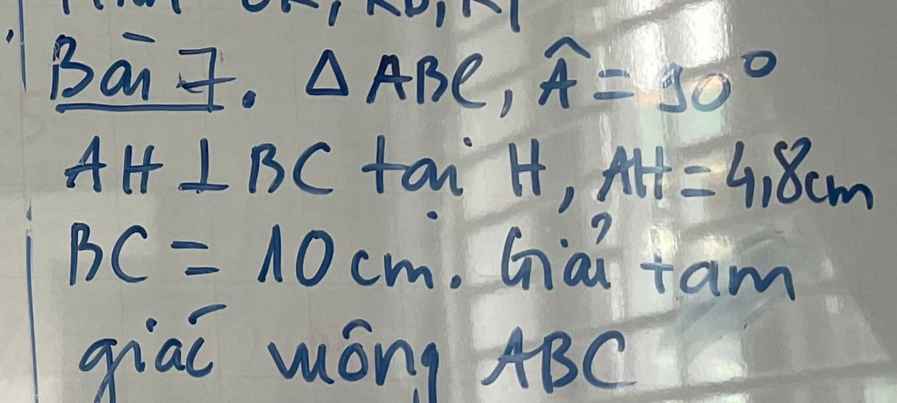Bào 7:
Áp dụng hệ thức lượng trong tam giác vuông có:
\(cos\widehat{BAH}=\dfrac{AH}{AB}=\dfrac{3}{7}\)\(\Rightarrow AH=\dfrac{3AB}{7}\)
\(AB^2+AC^2=BC^2=196\) \(\Leftrightarrow AB^2=196-AC^2\)
\(\dfrac{1}{AB^2}+\dfrac{1}{AC^2}=\dfrac{1}{AH^2}\)
\(\Leftrightarrow\dfrac{1}{196-AC^2}+\dfrac{1}{AC^2}=\dfrac{1}{\dfrac{9}{49}AB^2}\)
\(\Leftrightarrow\dfrac{1}{196-AC^2}+\dfrac{1}{AC^2}=\dfrac{49}{9\left(196-AC^2\right)}\)
\(\Leftrightarrow\dfrac{9AC^2}{9AC^2\left(196-AC^2\right)}+\dfrac{9\left(196-AC^2\right)}{9AC^2\left(196-AC^2\right)}=\dfrac{49AC^2}{9AC^2\left(196-AC^2\right)}\)
\(\Rightarrow9AC^2+9\left(196-AC^2\right)=49AC^2\)
\(\Leftrightarrow AC^2=36\) =>AC=6
Vậy AC=6 cm
Em cần giúp câu c và d ạ, mn giúp em với em đang cần gấp
