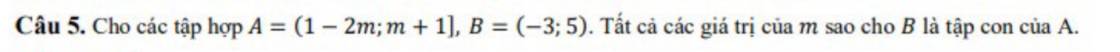\(\sqrt{x^2-x+2m-1}=\sqrt{x-3}\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge3\\x^2-x+2m-1=x-3\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge3\\f\left(x\right)=x^2-2x+2m+2=0\left(1\right)\end{matrix}\right.\)
Pt có đúng 2 nghiệm pb khi (1) có 2 nghiệm pb thỏa mãn \(3\le x_1< x_2\)
\(\Leftrightarrow\left\{{}\begin{matrix}\Delta'=1-\left(2m+2\right)>0\\f\left(3\right)=5+2m\ge0\\\dfrac{x_1+x_2}{2}=1>3\left(ktm\right)\end{matrix}\right.\)
Vậy ko tồn tại m thỏa mãn yêu cầu

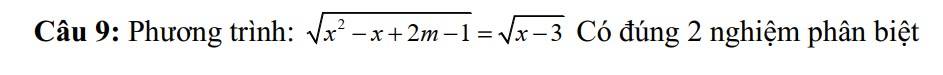 Giúp vớiiii
Giúp vớiiii


 GIÚP TUII VỚIIII! CẢM ƠN NHEEEEE
GIÚP TUII VỚIIII! CẢM ƠN NHEEEEE