(\(x\) - 2023)\(x-2024\) = 1
⇒ \(\left[{}\begin{matrix}x\ne2023;x-2024=0\\x-2023=1\end{matrix}\right.\)
⇒ \(\left[{}\begin{matrix}x=2024\\x=2024\end{matrix}\right.\)
Vậy \(x=2024\)
(x - 2023)ˣ⁻²⁰²⁴ = 1
(x - 2023)ˣ⁻²⁰²⁴ = (x - 2023)⁰ (x ≠ 2023)
x - 2024 = 0
x = 2024 (nhận)
Vậy x = 2024

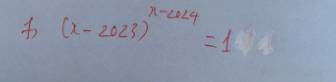





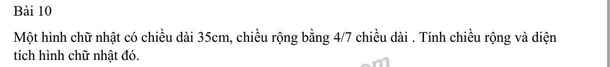

 Mọi người giúp mik đi mik cần gấp còn 20p nữa thôi ạ
Mọi người giúp mik đi mik cần gấp còn 20p nữa thôi ạ




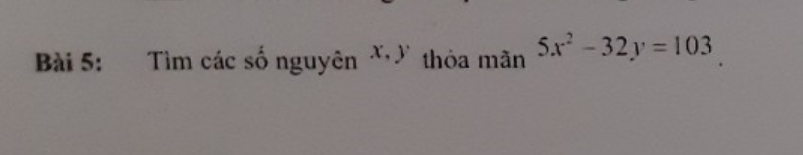

 giúp mik với mọi người, mik đang cần gấp, Thanks!
giúp mik với mọi người, mik đang cần gấp, Thanks!
