Ta có \(\widehat{A}+\widehat{B}+\widehat{C}=180^o\left(1\right)\)
\(\widehat{A}+2\widehat{B}=100^o\left(2\right)\)
\(=>\left(1\right)-\left(2\right)=>-\widehat{B}+\widehat{C}=180^o-100^o=>\widehat{C}-\widehat{B}=80^o\)
Theo giả thiết, ta có: \(\widehat{A}+2\widehat{B}=100^o\Rightarrow\widehat{A}+\widehat{B}=100^o-\widehat{B}\)
Xét tam giác ABC có: \(\widehat{A}+\widehat{B}+\widehat{C}=180^o\) (định lí)
\(100^o-\widehat{B}+\widehat{C}=180^o\) (vì \(\widehat{A}+\widehat{B}=100^o-\widehat{B}\))
\(\widehat{C}-\widehat{B}=80^o\)










 giúp em với ạ em cảm ơn trước ạ
giúp em với ạ em cảm ơn trước ạ


 giúp em với ạ em đang cần gấp lắm ạ em cảm ơn trước
giúp em với ạ em đang cần gấp lắm ạ em cảm ơn trước

 giúp em với ạ ai làm đc thì em xin cảm ơn ạ em đang cần gấp được ko ạ
giúp em với ạ ai làm đc thì em xin cảm ơn ạ em đang cần gấp được ko ạ
 MNG GIÚP EM BÀI 2 VỚI Ạ EM CẢM ƠN MNG NHIỀU Ạ
MNG GIÚP EM BÀI 2 VỚI Ạ EM CẢM ƠN MNG NHIỀU Ạ


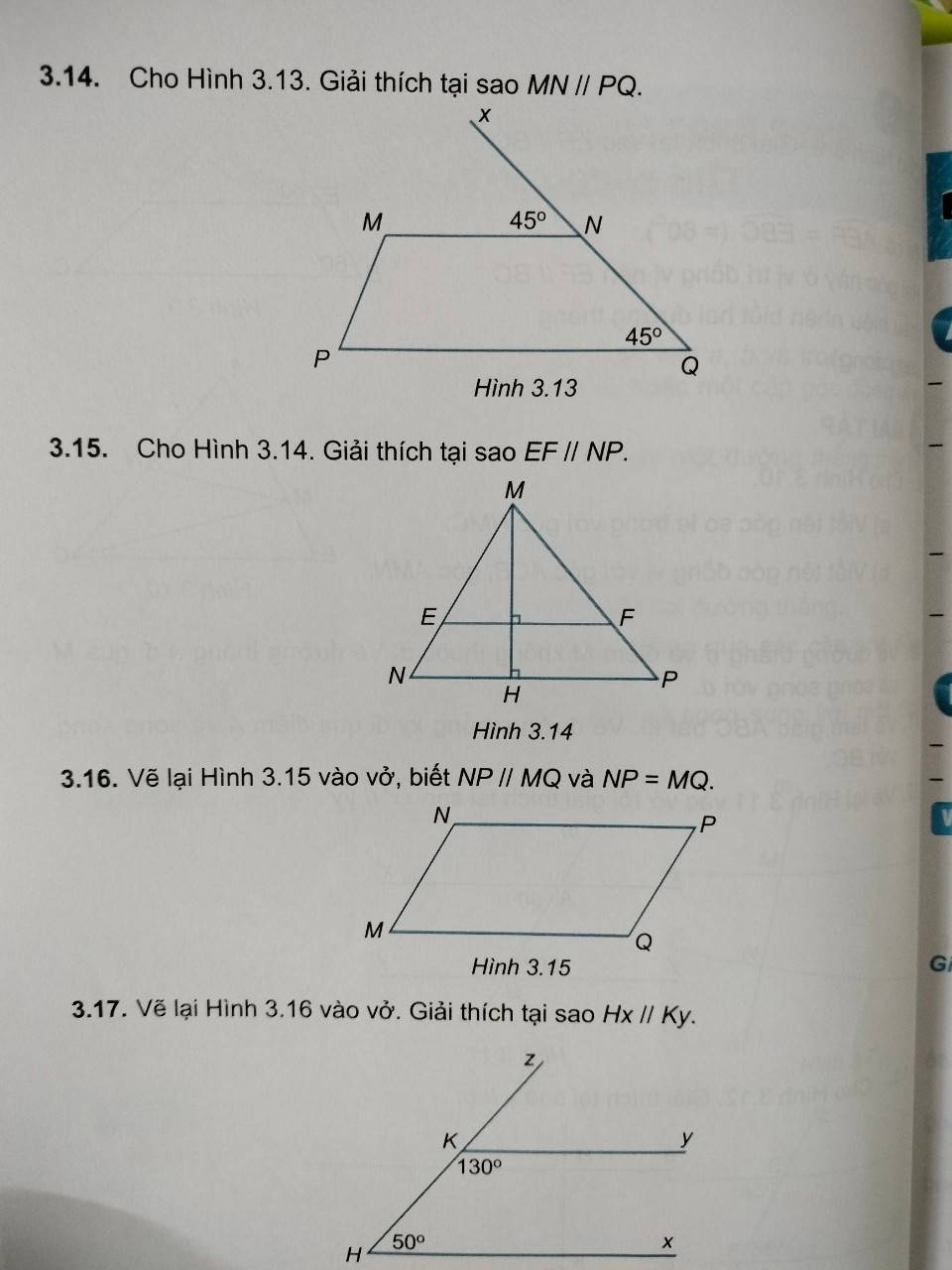 giúp em vài bài này với em đang cần gấp ạ em cảm ơn mn rất nhiều ạ
giúp em vài bài này với em đang cần gấp ạ em cảm ơn mn rất nhiều ạ