Question

Giúp em đề này với mn ơi

Answer 1

Bài 4:

a: Xét ΔADB vuông tại D và ΔAEC vuông tại E có

\(\widehat{DAB}\) chung

Do đó: ΔADB~ΔAEC

b: Xét ΔHEB vuông tại E và ΔHDC vuông tại D có

\(\widehat{EHB}=\widehat{DHC}\)(hai góc đối đỉnh)

Do đó: ΔHEB~ΔHDC

=>\(\dfrac{HE}{HD}=\dfrac{HB}{HC}\)

=>\(HE\cdot HC=HB\cdot HD\)

Bài 3:

a: \(x^6-7x^3-8=0\)

=>\(x^6-8x^3+x^3-8=0\)

=>\(\left(x^3-8\right)\left(x^3+1\right)=0\)

=>\(\left[{}\begin{matrix}x^3=8\\x^3=-1\end{matrix}\right.\)

=>\(\left[{}\begin{matrix}x=2\\x=-1\end{matrix}\right.\)

Bài 2:

a: \(\left(6x+7\right)\left(2x-3\right)-\left(4x+1\right)\left(3x-\dfrac{7}{4}\right)\)

\(=12x^2-18x+14x-21-\left(12x^2-7x+3x-\dfrac{7}{4}\right)\)

\(=12x^2-4x-21-12x^2+4x+\dfrac{7}{4}=-21+1,75=-19,25\)

b: \(x^2-2y^2=xy\)

=>\(x^2-xy-2y^2=0\)

=>(x-2y)(x+y)=0

mà x+y<>0

nên x-2y=0

=>x=2y

\(P=\dfrac{x-y}{x+y}=\dfrac{2y-y}{2y+y}=\dfrac{1}{3}\)

Bài 1:

a: ĐKXĐ: \(x\notin\left\{\dfrac{1}{2};1;-1\right\}\)

\(A=\left(\dfrac{1}{1-x}+\dfrac{2}{x+1}-\dfrac{5-x}{1-x^2}\right):\dfrac{1-2x}{x^2-1}\)

\(=\left(\dfrac{-1}{x-1}+\dfrac{2}{x+1}-\dfrac{x-5}{\left(x-1\right)\left(x+1\right)}\right)\cdot\dfrac{\left(x-1\right)\left(x+1\right)}{-2x+1}\)

\(=\dfrac{-x-1+2x-2-x+5}{\left(x-1\right)\left(x+1\right)}\cdot\dfrac{\left(x-1\right)\left(x+1\right)}{-2x+1}\)

\(=\dfrac{2}{-2x+1}\)

b: Để A>0 thì \(\dfrac{2}{-2x+1}>0\)

=>-2x+1>0

=>-2x>-1

=>\(x< \dfrac{1}{2}\)

Kết hợp ĐKXĐ, ta được: \(\left\{{}\begin{matrix}x< \dfrac{1}{2}\\x\ne-1\end{matrix}\right.\)
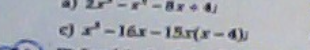