a) Hàm số trên nghịch biến trên R vì:
\(1< \sqrt{5}\Rightarrow1-\sqrt{5}< 0\)
\(\Rightarrow\) hệ số \(a< 0\)
b) Khi \(x=1+\sqrt{5}\)
\(y=\left(1-\sqrt{5}\right)\left(1+\sqrt{5}\right)-1\)
\(y=1^2-\left(\sqrt{5}\right)^2-1\)
\(y=1-5-1\)
\(y=-5\)
c) Khi \(y=\sqrt{5}\) khi và chỉ khi:
\(\left(1-\sqrt{5}\right)x-1=\sqrt{5}\)
\(\Leftrightarrow\left(1-\sqrt{5}\right)x=1+\sqrt{5}\)
\(\Leftrightarrow x=\dfrac{1+\sqrt{5}}{1-\sqrt{5}}\)
\(\Leftrightarrow x=\dfrac{\left(1+\sqrt{5}\right)^2}{1-5}\)
\(\Leftrightarrow x=-\dfrac{3+\sqrt{5}}{2}\)

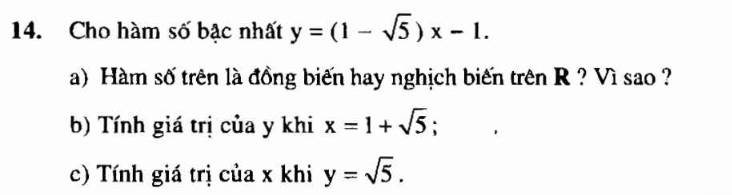 giúp e với aa, e cảm ơn nhiềuu
giúp e với aa, e cảm ơn nhiềuu





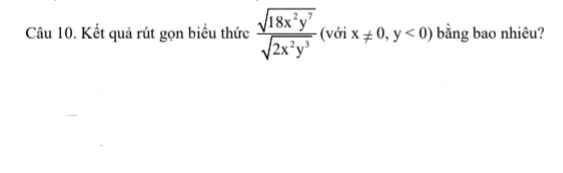
 giúp e với aa, e cảm ơnn
giúp e với aa, e cảm ơnn giúp e với aaa, e cảm ơn
giúp e với aaa, e cảm ơn giúp e với a, e cảm ơn
giúp e với a, e cảm ơn
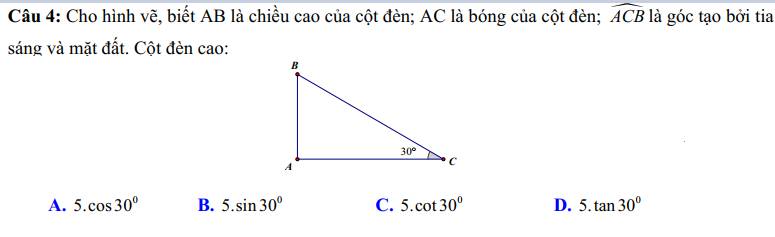
 Mn giúp e với
Mn giúp e với 

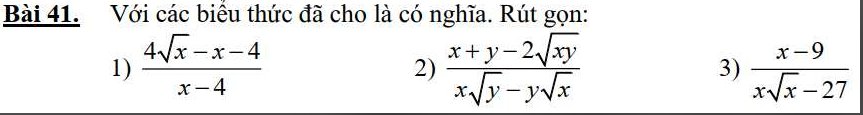 giúp e gấp với ạ, e cảm ơn
giúp e gấp với ạ, e cảm ơn