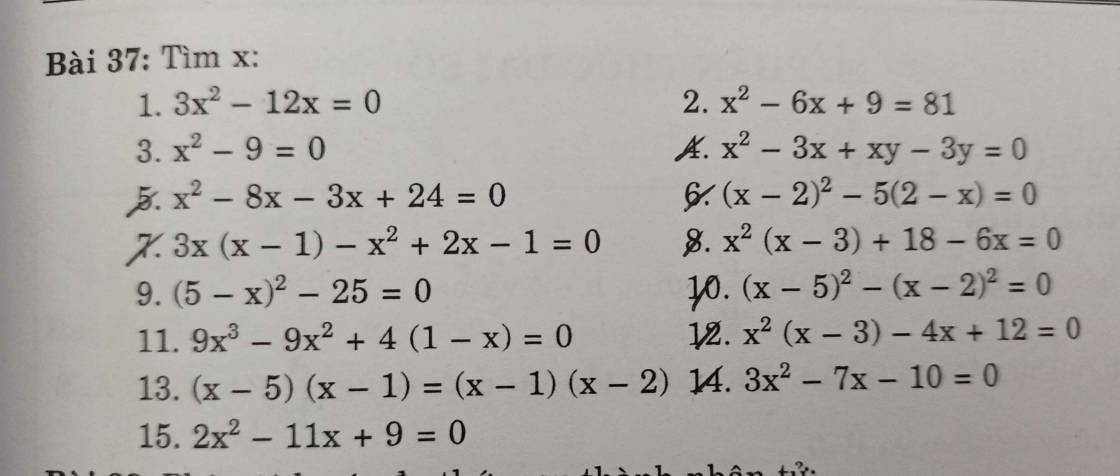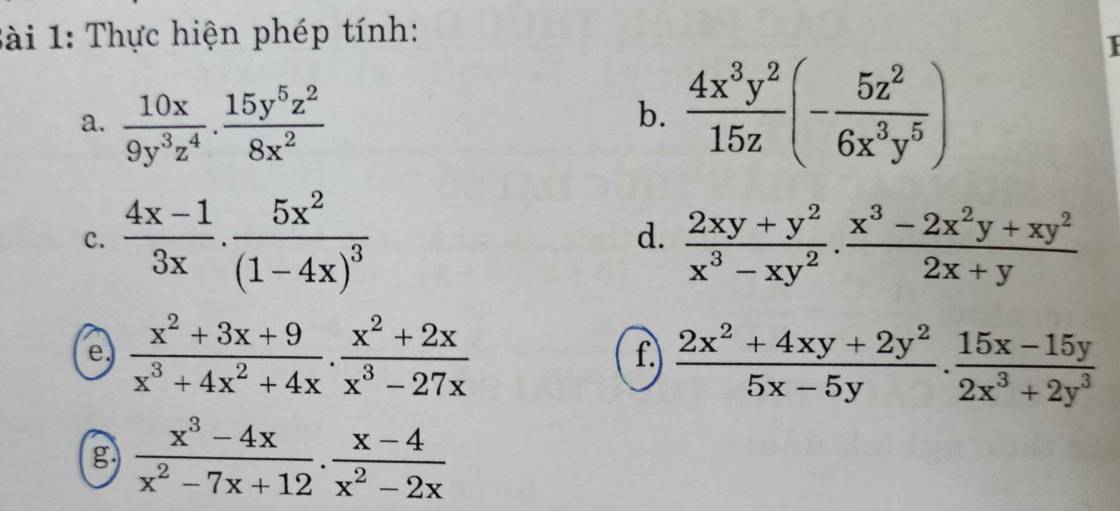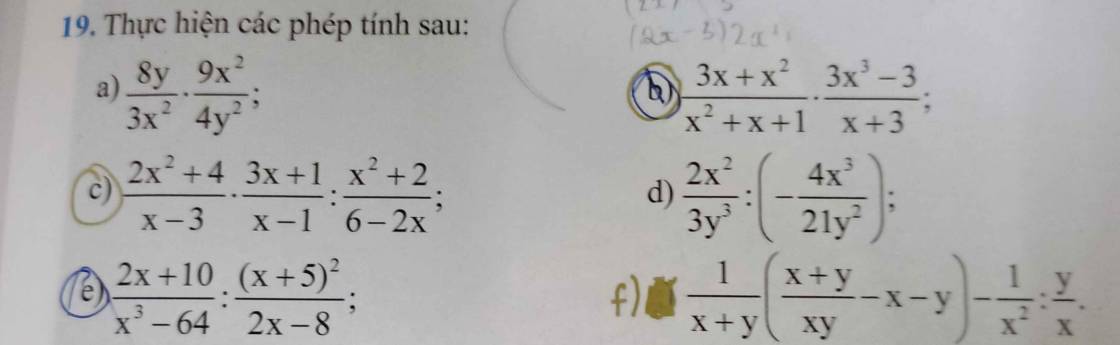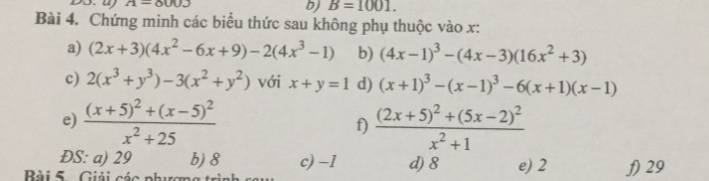b) ĐKXĐ: x ≠ 2; x ≠ 4
Phương trình đã cho tương đương:
(x - 3)(x - 4) + (x - 2)² = -(x - 2)(x - 4)
x² - 4x - 3x + 12 + x² - 4x + 4 = -x² + 4x + 2x - 8
2x² + x² - 11x - 6x + 16 + 8 = 0
3x² - 17x + 24 = 0
3x² - 9x - 8x + 24 = 0
(3x² - 9x) - (8x - 24) = 0
3x(x - 3) - 8(x - 3) = 0
(x - 3)(3x - 8) = 0
x - 3 = 0 hoặc 3x - 8 = 0
*) x - 3 = 0
x = 3 (nhận)
3x - 8 = 0
3x = 8
x = 8/3 (nhận)
Vậy S = {8/3; 3}
c) ĐKXĐ: x ≠ -3/4; x ≠ -5
Phương trình đã cho tương đương:
x³ - (x - 1)³ = (7x - 1)(x + 5) - x(4x + 3)
x³ - x³ + 3x² - 3x + 1 = 7x² + 35x - x - 5 - 4x² - 3x
3x² - 7x² + 4x² - 3x - 31x = -5 - 1
-34x = -6
x = -2/17 (nhận)
Vậy S = {-2/17}
d) \(\dfrac{2}{x-1}+\dfrac{2x+3}{x^2+x+1}=\dfrac{\left(2x+1\right)\left(2x-1\right)}{x^3-1}\left(x\ne1\right)\)
\(\Leftrightarrow\dfrac{2\left(x^2+x+1\right)}{\left(x-1\right)\left(x^2+x+1\right)}+\dfrac{\left(2x+3\right)\left(x-1\right)}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{\left(2x\right)^2-1^2}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(\Leftrightarrow2\left(x^2+x+1\right)+\left(2x+3\right)\left(x-1\right)=4x^2-1\)
\(\Leftrightarrow2x^2+2x+2+2x^2-2x+3x-3=4x^2-1\)
\(\Leftrightarrow4x^2-x-1=4x^2-1\)
\(\Leftrightarrow-x-1=-1\)
\(\Leftrightarrow-x=0\)
\(\Leftrightarrow x=0\left(tm\right)\)
e) \(\dfrac{x+2}{x+1}+\dfrac{3}{x-2}=\dfrac{3}{x^2-x-2}+1\left(x\ne-1;x\ne2\right)\)
\(\Leftrightarrow\dfrac{\left(x+2\right)\left(x-2\right)}{\left(x+1\right)\left(x-2\right)}+\dfrac{3\left(x+1\right)}{\left(x+1\right)\left(x-2\right)}=\dfrac{3}{\left(x+1\right)\left(x-2\right)}+\dfrac{\left(x+1\right)\left(x-2\right)}{\left(x+1\right)\left(x-2\right)}\)
\(\Leftrightarrow\left(x+2\right)\left(x-2\right)+3\left(x+1\right)=3+\left(x+1\right)\left(x-2\right)\)
\(\Leftrightarrow x^2-4+3x+3=3+x^2-x-2\)
\(\Leftrightarrow x^2+3x-1=x^2-x+1\)
\(\Leftrightarrow3x-1=-x+1\)
\(\Leftrightarrow3x+x=1+1\)
\(\Leftrightarrow4x=2\)
\(\Leftrightarrow x=\dfrac{1}{2}\) (tm)