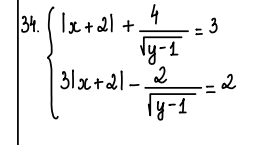Đặt \(\left\{{}\begin{matrix}a=\left|x+2\right|\ge0\\b=\dfrac{1}{\sqrt{y-1}}>0\end{matrix}\right.\)
Hpt \(\Leftrightarrow\left\{{}\begin{matrix}a+4b=3\\3a-2b=2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a+4b=3\\6a-4b=4\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a+4b=3\\7a=7\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}a=1\left(tm\right)\\b=\dfrac{1}{2}\left(tm\right)\end{matrix}\right.\)
- Với \(a=1\Leftrightarrow\left|x+2\right|=1\Leftrightarrow\left[{}\begin{matrix}x+2=1\\x+2=-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-3\end{matrix}\right.\)
- Với \(b=\dfrac{1}{2}\Leftrightarrow\dfrac{1}{\sqrt{y-1}}=\dfrac{1}{2}\Leftrightarrow\sqrt{y-1}=2\Leftrightarrow y-1=4\Leftrightarrow y=5\)
Vậy \(\left(x;y\right)=\left(-1;5\right);\left(-3;5\right)\)