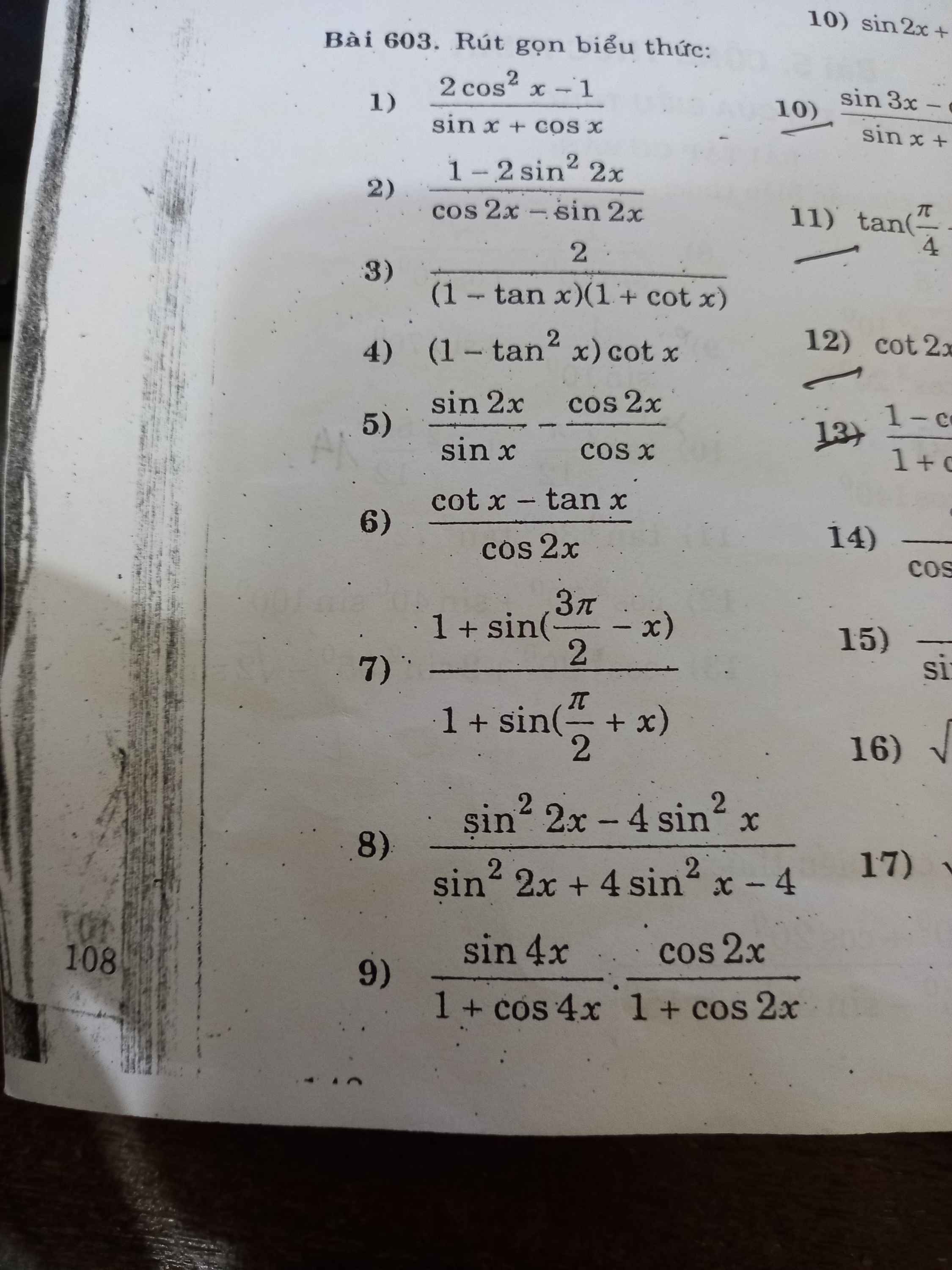g) ĐKXĐ: \(x\ge\dfrac{3}{2}\)
\(\Leftrightarrow\sqrt{2x-3}=9-x\\ \Leftrightarrow2x-3=81-18x+x^2\left(x\le9\right)\\ \Leftrightarrow x^2-20x+84=0\\ \Leftrightarrow\left(x^2-14x\right)-\left(6x-84\right)=0\\ \Leftrightarrow x\left(x-14\right)-6\left(x-14\right)=0\\ \Leftrightarrow\left(x-6\right)\left(x-14\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=6\left(tm\right)\\x=14\left(ktm\right)\end{matrix}\right.\)
Vậy \(x=6\)
h) \(ĐKXĐ:x\ge\dfrac{1}{4}\)
\(\Leftrightarrow2x-5=16x^2-8x+1\\ \Leftrightarrow16x^2-10x+6=0\\ \Leftrightarrow8x^2-5x+3=0\\ \Leftrightarrow\left(4x^2-5x+\dfrac{25}{16}\right)+x^2+\dfrac{23}{16}=0\\ \Leftrightarrow\left(2x-\dfrac{5}{4}\right)^2+x^2+\dfrac{23}{16}=0\left(vôlí\right)\)
Vậy pt trên vô nghiệm