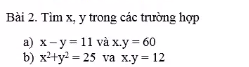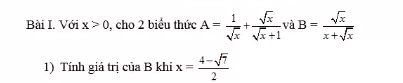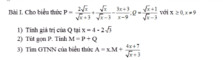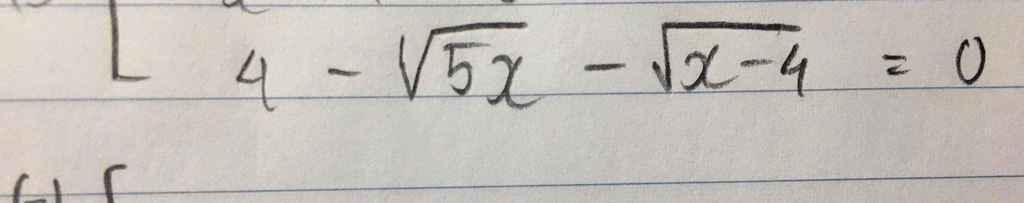a: ĐKXĐ: \(\left\{{}\begin{matrix}x>0\\x\ne1\end{matrix}\right.\)
\(A=\left(\dfrac{1}{x-\sqrt{x}}+\dfrac{1}{\sqrt{x}-1}\right):\dfrac{\sqrt{x}+1}{\left(\sqrt{x}-1\right)^2}\)
\(=\left(\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}+\dfrac{\sqrt{x}}{\sqrt{x}\left(\sqrt{x}-1\right)}\right)\cdot\dfrac{\left(\sqrt{x}-1\right)^2}{\sqrt{x}+1}\)
\(=\dfrac{\sqrt{x}+1}{\sqrt{x}+1}\cdot\dfrac{\left(\sqrt{x}-1\right)^2}{\sqrt{x}\left(\sqrt{x}-1\right)}=\dfrac{\sqrt{x}-1}{\sqrt{x}}\)
b: \(P=A-9\sqrt{x}=\dfrac{\sqrt{x}-1-9x}{\sqrt{x}}=\dfrac{-9x+\sqrt{x}-1}{\sqrt{x}}\)
\(=-9\sqrt{x}+1-\dfrac{1}{\sqrt{x}}=-\left(9\sqrt{x}+\dfrac{1}{\sqrt{x}}\right)+1\)
\(9\sqrt{x}+\dfrac{1}{\sqrt{x}}>=2\cdot\sqrt{9\sqrt{x}\cdot\dfrac{1}{\sqrt{x}}}=6\) với mọi x thỏa mãn ĐKXĐ
=>\(-\left(9\sqrt{x}+\dfrac{1}{\sqrt{x}}\right)< =-6\) với mọi x thỏa mãn ĐKXĐ
=>\(P=-\left(9\sqrt{x}+\dfrac{1}{\sqrt{x}}\right)+1< =-5\forall x\) thỏa mãn ĐKXĐ
Dấu '=' xảy ra khi \(9\sqrt{x}=\dfrac{1}{\sqrt{x}}\)
=>9x=1
=>\(x=\dfrac{1}{9}\)(nhận)






 giải giúp mình với ạa , mình cảm ơn.
giải giúp mình với ạa , mình cảm ơn.