1: Thay x=9 vào A, ta được:
\(A=\dfrac{3-1}{3+1}=\dfrac{2}{4}=\dfrac{1}{2}\)
2: \(B=\dfrac{\sqrt{x}-2}{\sqrt{x}}+\dfrac{1}{\sqrt{x}-1}-\dfrac{2}{x-\sqrt{x}}\)
\(=\dfrac{\sqrt{x}-2}{\sqrt{x}}+\dfrac{1}{\sqrt{x}-1}-\dfrac{2}{\sqrt{x}\left(\sqrt{x}-1\right)}\)
\(=\dfrac{\left(\sqrt{x}-2\right)\left(\sqrt{x}-1\right)+\sqrt{x}-2}{\left(\sqrt{x}-1\right)\cdot\sqrt{x}}\)
\(=\dfrac{\sqrt{x}\left(\sqrt{x}-2\right)}{\sqrt{x}\left(\sqrt{x}-1\right)}=\dfrac{\sqrt{x}-2}{\sqrt{x}-1}\)
3: \(P=A\cdot B=\dfrac{\sqrt{x}-2}{\sqrt{x}-1}\cdot\dfrac{\sqrt{x}-1}{\sqrt{x}+1}=\dfrac{\sqrt{x}-2}{\sqrt{x}+1}\)
\(\sqrt{P}< \dfrac{1}{2}\)
=>\(0< =P< \dfrac{1}{4}\)
=>\(\left\{{}\begin{matrix}\dfrac{\sqrt{x}-2}{\sqrt{x}+1}>=0\\\dfrac{\sqrt{x}-2}{\sqrt{x}+1}< \dfrac{1}{4}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=4\\\dfrac{\sqrt{x}-2}{\sqrt{x}+1}-\dfrac{1}{4}< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>=4\\\dfrac{4\left(\sqrt{x}-2\right)-\sqrt{x}-1}{4\left(\sqrt{x}+1\right)}< 0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=4\\3\sqrt{x}-9< 0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=4\\0< =x< 9\end{matrix}\right.\Leftrightarrow4< =x< 9\)

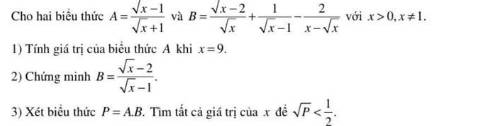



 cíu tui cíu tui
cíu tui cíu tui cíu tui cíu tui
cíu tui cíu tui

 cíu tui cíu tui
cíu tui cíu tui

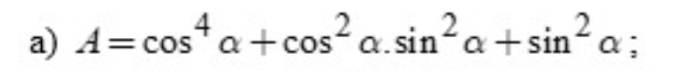
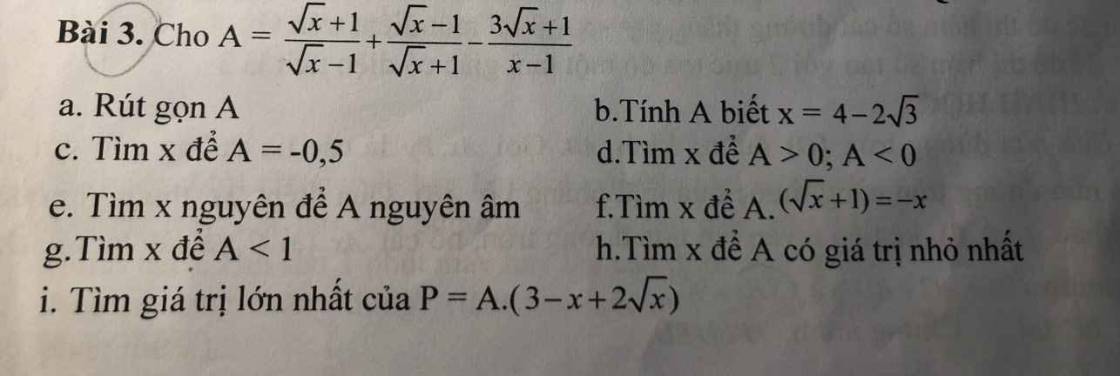 cíu cíu
cíu cíu cíu lẹ cíu lẹ
cíu lẹ cíu lẹ