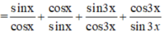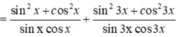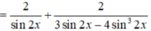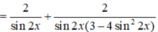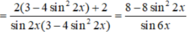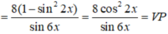Các câu hỏi tương tự
cho mình hỏi: chứng minh đẳng thức này: \(\sin^2x\left(1+\cot x\right)x+\cos^2\left(1+\tan x\right)=\left(\sin x+\cos x\right)^2\)có thể giải bằng cách lấy VT - VP = 0 có dc ko và tại sao ?
chứng minh đẳng thức này \(\frac{\sin x+\cos x-1}{\sin x-\cos x+1}=\frac{\cos x}{1+\sin x}\) có thể quy đồng rồi lấy VT - VP = 0 có dc ko và tại sao ?
Thanks nhiều
Chứng minh rằng:
\(\frac{sin\left(x\right)+sin\left(\frac{x}{2}\right)}{1+cos\left(x\right)+cos\left(\frac{x}{2}\right)}=tan\left(\frac{x}{2}\right)\)
CMR:
a, \(\frac{\cot^2x-\sin^2x}{\cot^2x-tan^2x}=sin^2x.\cos^2x\)
b, \(\frac{\tan x}{1-\tan^2x}.\frac{\cot^2-1}{\cot x}=1\)
c, \(\frac{1+\sin x.\cos x}{\sin^2x-\cos^2x}=\frac{\tan x+1}{\cot x+1}\)
d, \(\frac{\sin x+\cos x-1}{\sin x-cosx+1}=\frac{\cos x}{1+sinx}\)
Chứng minh đẳng thức: (tan x /1 - tan^2 x) (cot^2 x - 1/cot x) = 1
Xem chi tiết
Tìm các giá trị lượng giác còn lại biết:
a) Cho sin \(x=-\dfrac{4}{5}\)và \(90^o< x< 180^o\)
b) Cho \(\sin x=\dfrac{\sqrt{3}}{2}\)và \(270^o< x< 360^o\)
c) Cho \(\cos x=-\dfrac{1}{3}\)và \(0^o< x< 90^o\)
Tính giá trị của biểu thức sau : B= \(\dfrac{tan\left(\dfrac{21\pi}{2}-x\right).cos\left(38\pi-x\right).sin\left(x-7\pi\right)}{sin\left(\dfrac{13\pi}{2}-x\right).cos\left(x-2023\pi\right)}\)
Tính giá trị của biểu thức sau: B= \(\dfrac{tan\left(\dfrac{23\pi}{2}+x\right).sin\left(2022\pi-x\right).cos\left(x-2021\pi\right)}{cos\left(\dfrac{2021\pi}{2}-x\right).sin\left(x+2023\pi\right)}\)
Giải các pt
a) \(\sqrt{2}\sin\left(2x+\dfrac{\pi}{4}\right)=3\sin x+\cos x+2\)
b) \(\dfrac{\left(2-\sqrt{3}\right)\cos x-2\sin^2\left(\dfrac{x}{2}-\dfrac{\pi}{4}\right)}{2\cos x-1}=1\)
c) \(2\sqrt{2}\cos\left(\dfrac{5\pi}{12}-x\right)\sin x=1\)
Cho tam giác ABC. Chứng minh \(\dfrac{\sin^3\dfrac{B}{2}}{\cos\left(\dfrac{A+C}{2}\right)}\)+ \(\dfrac{\cos^3\dfrac{B}{2}}{sin\left(\dfrac{A+C}{2}\right)}\)-\(\dfrac{\cos\left(A-C\right)}{\sin B}\).\(\tan B=2\)
cứuuuuuuu
\(a) A = a %2 sin 90 ^∘ + b ^2 cos 90 ^∘ + c ^2 cos 180 ^∘\)
\(b) B = 3 − sin ^2 90 ^∘ + 2 cos ^2 60 ^∘ − 3 tan ^2 45 ^∘\)
\(c) C = sin ^2 45 ^∘ − 2 sin ^2 50 ^∘ + 3 cos ^2 45 ^∘ − 2 sin ^2 40 ^∘ + 4 tan 55 ^∘ ⋅ tan 35 ^∘\)