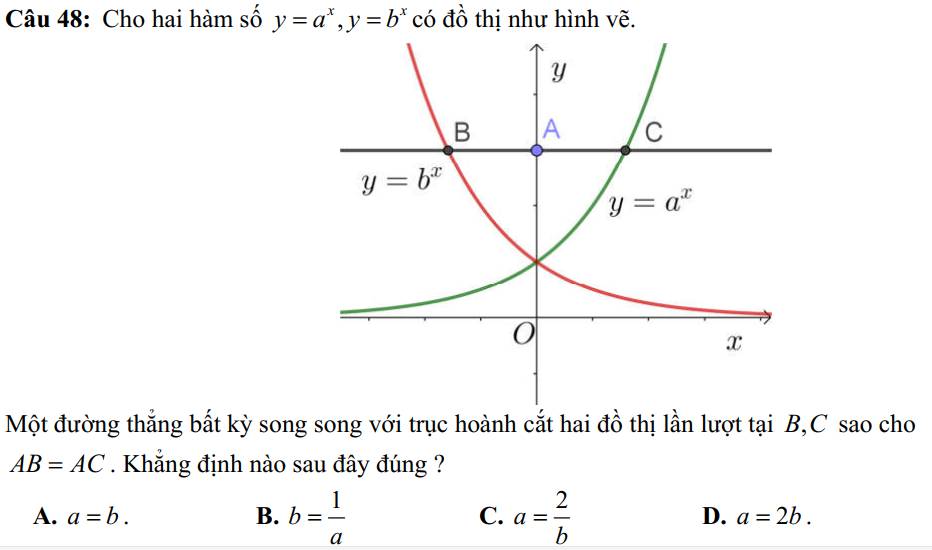
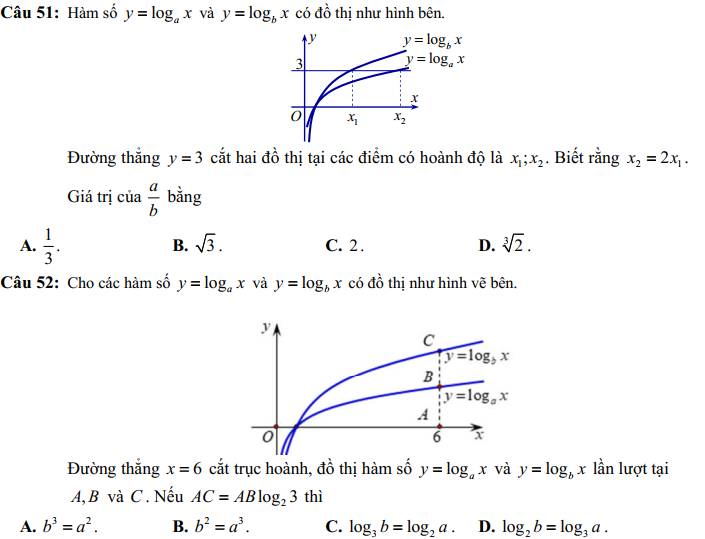
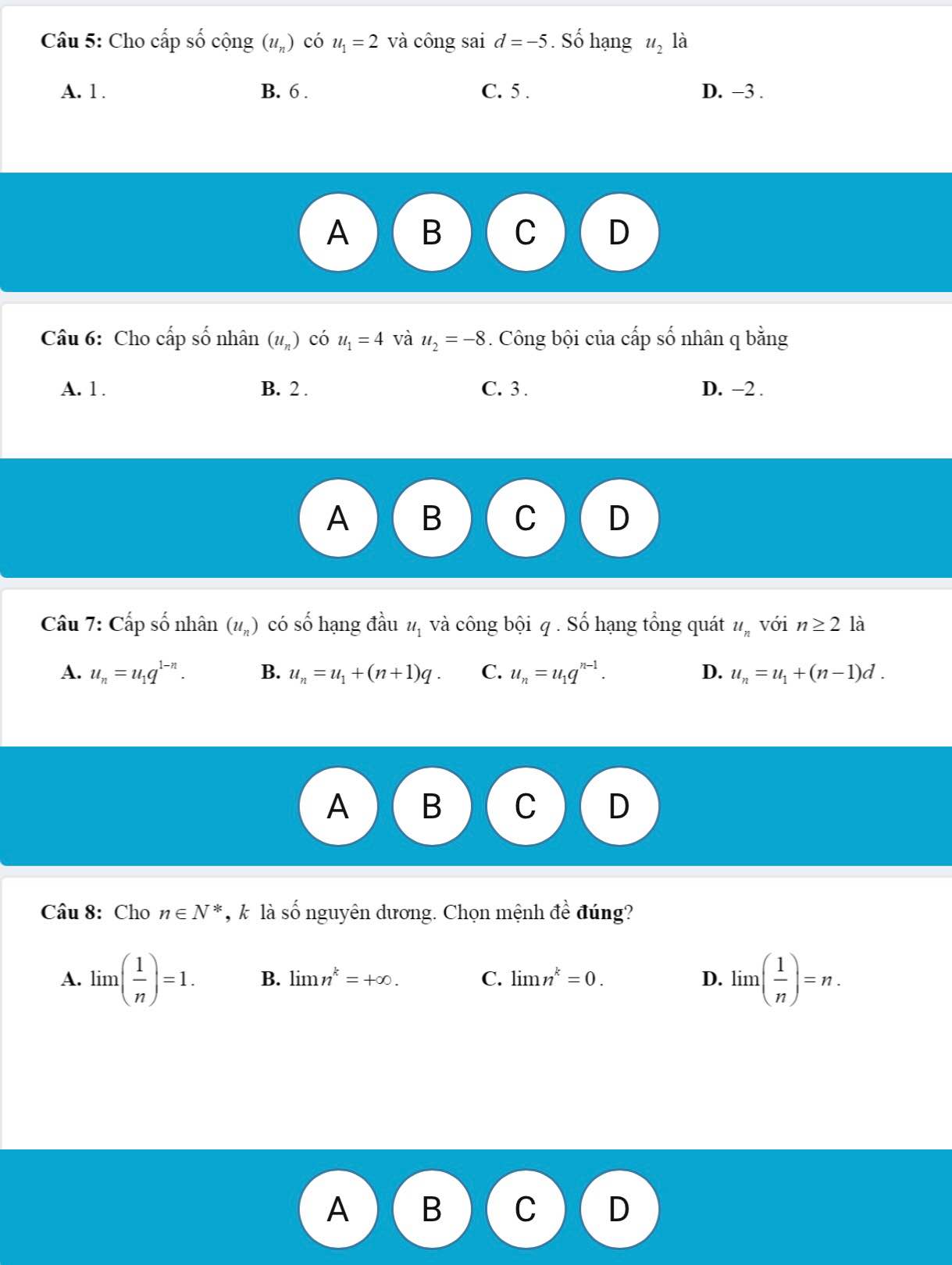
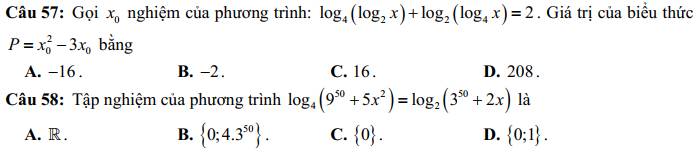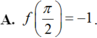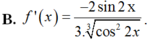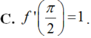51:
\(log_{25}\left(5^{x+1}-5\right)=log_{5^2}\left[5\left(5^x-1\right)\right]\)
\(=\dfrac{1}{2}\cdot log_5\left[5\left(5^x-1\right)\right]\)
\(=\dfrac{1}{2}\left(log_55+log_5\left(5^x-1\right)\right)\)
\(=\dfrac{1}{2}\left(1+t\right)\)
Phương trình sẽ trở thành:
\(t\cdot\dfrac{1}{2}\left(t+1\right)=1\)
=>\(t\left(t+1\right)=2\)
=>\(t^2+t-2=0\)
=>Chọn B
52:
ĐKXĐ: \(\left\{{}\begin{matrix}\sqrt{4-x}>0\\\left(4+x\right)^3>0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x< 4\\x>-4\end{matrix}\right.\)
=>-4<x<4
\(log_4\left(x+1\right)^2+2=log_{\sqrt{2}}\sqrt{4-x}+log_8\left(4+x\right)^3\)
=>\(2\cdot\dfrac{1}{2}\cdot log_2\left(x+1\right)+2=log_{2^{\dfrac{1}{2}}}\left(4-x\right)^{\dfrac{1}{2}}+log_{2^3}\left(4+x\right)^3\)
=>\(log_2\left(x+1\right)+2=log_2\left(4-x\right)+log_2\left(x+4\right)\)
=>\(log_2\left(4x+4\right)=log_2\left[\left(4-x\right)\left(x+4\right)\right]\)
=>\(4x+4=\left(4-x\right)\left(x+4\right)\)
=>\(4x+4=16-x^2\)
=>\(4x+4-16+x^2=0\)
=>\(x^2+4x-12=0\)
=>(x+6)(x-2)=0
=>\(\left[{}\begin{matrix}x=-6\left(loại\right)\\x=2\left(nhận\right)\end{matrix}\right.\)
=>Chọn B
Câu 53:
ĐKXĐ: \(x>0\)
\(\dfrac{1}{2}log_{\sqrt{3}}\left(x+3\right)+\dfrac{1}{2}log_9\left(x-1\right)^4=2\cdot log_94x\)
=>\(\dfrac{1}{2}\cdot log_{3^{\dfrac{1}{2}}}\left(x+3\right)+\dfrac{1}{2}\cdot log_{3^2}\left(x-1\right)^4=2\cdot log_{3^2}4x\)
=>\(log_3\left(x+3\right)+log_3\left(x-1\right)=log_34x\)
=>\(log_3\left[\left(x+3\right)\left(x-1\right)\right]=log_34x\)
=>\(\left(x+3\right)\left(x-1\right)=4x\)
=>\(x^2+2x-3-4x=0\)
=>\(x^2-2x-3=0\)
=>(x-3)(x+1)=0
=>\(\left[{}\begin{matrix}x=3\left(nhận\right)\\x=-1\left(loại\right)\end{matrix}\right.\)
=>Chọn B
Câu 54:
\(2\cdot log_2\left(2x-2\right)+log_2\left(x-3\right)^2=2\)
=>\(2\cdot log_2\left(2x-2\right)+2\cdot log_2\left(x-3\right)=2\)
=>\(log_2\left(2x-2\right)+log_2\left(x-3\right)=1\)
=>\(log_2\left[\left(2x-2\right)\left(x-3\right)\right]=1\)
=>\(\left(2x-2\right)\cdot\left(x-3\right)=2\)
=>\(2x^2-6x-2x+6=2\)
=>\(2x^2-8x+4=0\)
=>\(x^2-4x+2=0\)
=>\(\left[{}\begin{matrix}x=2+\sqrt{2}\left(nhận\right)\\x=2-\sqrt{2}\left(loại\right)\end{matrix}\right.\)
=>Xem lại đề nha