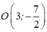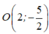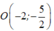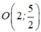Question

cho 3 điểm A(2;3), B(-1;-1), c(-3;1)
tìm tọa độ diểm D để tứ giác ABCD là hình bình hành. Tìm tọa độ tâm  I của hình bình hành ABCD

Answer 1

A(2;3); B(-1;-1); C(-3;1); D(x;y)

\(\overrightarrow{AB}=\left(-3;-4\right);\overrightarrow{DC}=\left(-3-x;1-y\right)\)

ta có: ABCD là hình bình hành

=>\(\overrightarrow{AB}=\overrightarrow{DC}\)

=>\(\left\{{}\begin{matrix}-3-x=-3\\1-y=-4\end{matrix}\right.\)

=>\(\left\{{}\begin{matrix}x+3=3\\y-1=4\end{matrix}\right.\)

=>\(\left\{{}\begin{matrix}x=0\\y=5\end{matrix}\right.\)

Vậy: D(0;5)

Tọa độ tâm I của hình bình hành ABCD là:

\(\left\{{}\begin{matrix}x_I=\dfrac{x_A+x_C}{2}=\dfrac{2+\left(-3\right)}{2}=-\dfrac{1}{2}\\y_I=\dfrac{3+1}{2}=\dfrac{4}{2}=2\end{matrix}\right.\)

vậy: I(-0,5;2)