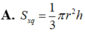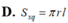Để hàm số `g(x)` nghịch biến trên `(e;e^2024)` thì:
`g'(x) = 1/x f'(lnx) - 2mx +4m <0 forall x in (e;e^2024)`
`<=> 1/x . (lnx+1).e^(lnx) -2mx+4m<0 forall x in (e;e^2024)`
`<=>(lnx+1) -2mx+4m<0 forall x in (e;e^2024)`
`<=>(lnx+1) <m(2x+4) forall x in (e;e^2024)`
`<=> m > (lnx+1)/(2x+4) forall x in (e;e^2024)`
`<=> m > Max ((lnx+1)/(2x+4)) forall x in (e;e^2024)`
Xét hàm số `h(x)=(lnx+1)/(2x+4)` trên `(e;e^2024)`
\(h'\left(x\right)=\dfrac{\dfrac{1}{x}\left(2x+4\right)-2\left(lnx+1\right)}{\left(2x+4\right)^2}=\dfrac{\dfrac{4}{x}-2lnx}{\left(2x+4\right)^2}=\dfrac{4-2xlnx}{x\left(2x+4\right)^2}< 0\forall x\in\left(e;e^{2024}\right)\)
`=> h(x)` nghịch biến trên `(e;e^2024)`
`=>m > (lne +1)/(2e+4) `
`=> ` Có 2024 giá trị nguyên của m thỏa mãn