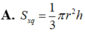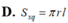Question

Answer 1

Đặt \(\dfrac{1}{x}=y\)

\(\Rightarrow y^3+e^y=\left(x+m\right)^3+e^{x+m}\) (1)

Xét hàm: \(f\left(t\right)=t^3+e^t\)

\(f'\left(t\right)=3t^2+e^t>0;\forall t\) nên \(f\left(t\right)\) đồng biến trên TXĐ

Nên (1) tương đương \(y=x+m\Leftrightarrow\dfrac{1}{x}=x+m\)

\(\Leftrightarrow\dfrac{1}{x}-x=m\)

Xét hàm \(f\left(x\right)=\dfrac{1}{x}-x\) ... lập BBT \(\Rightarrow\) pt có nghiệm với mọi m (ko chỉ có nghiệm mà luôn có hẳn 2 nghiệm)

Đoạn lập BBT của \(f\left(x\right)\) chắc em tự xử lý được, hàm này nghịch biến trên miền xác định.