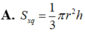\(\Leftrightarrow f\left(x^3.f\left(x\right)\right)=-1\)
Từ đồ thị ta được \(\left[{}\begin{matrix}x^3.f\left(x\right)=0\\x^3.f\left(x\right)=a\\x^3.f\left(x\right)=b\end{matrix}\right.\)
Xét \(x^3.f\left(x\right)=0\Leftrightarrow\left[{}\begin{matrix}x=0\\f\left(x\right)=0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=c\end{matrix}\right.\)
Xét \(x^3.f\left(x\right)=b\Leftrightarrow f\left(x\right)=\dfrac{b}{x^3}\)
Hàm \(y=\dfrac{b}{x^3}\) với \(b>0\) có dạng hypebol 2 nhánh tương tự \(y=\dfrac{1}{x}\) nên dễ dàng phác thảo được dạng đồ thị, nó sẽ cắt \(y=f\left(x\right)\) tại 2 điểm thỏa mãn \(x>c\) và \(x< 0\)
Hay pt \(x^3.f\left(x\right)=b\) có 2 nghiệm
Tương tự \(x^3.f\left(x\right)=c\) cũng có 2 nghiệm
Vậy pt đã cho có 6 nghiệm thực pb