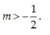Question

tìm tất cả các giá trị thực của tham số m để parabol y=x^2 -9|x| cắt đường thẳng y=m tại 4 đ pb

 

 

Answer 1

\(y=x^2-9\left|x\right|=\left[{}\begin{matrix}x^2-9x\left(x\ge0\right)\\x^2+9x\left(x< 0\right)\end{matrix}\right.\)

Theo đồ thị ta có hàm số \(y=x^2-9x\) đạt cực tiểu tại \(x=\dfrac{9}{2}\)

\(\Rightarrow y_{min}=\dfrac{81}{4}-9.\dfrac{9}{2}=-\dfrac{81}{4}\)

Đồ thị hàm số \(y=x^2+9x\) sẽ đối xứng qua trục \(Oy\)

\(\Rightarrow\) Để hàm số \(y=x^2-9\left|x\right|\) cắt đường thẳng \(y=m\) tại \(4\) điểm phân biệt

\(\Rightarrow-\dfrac{81}{4}< m< 0\)