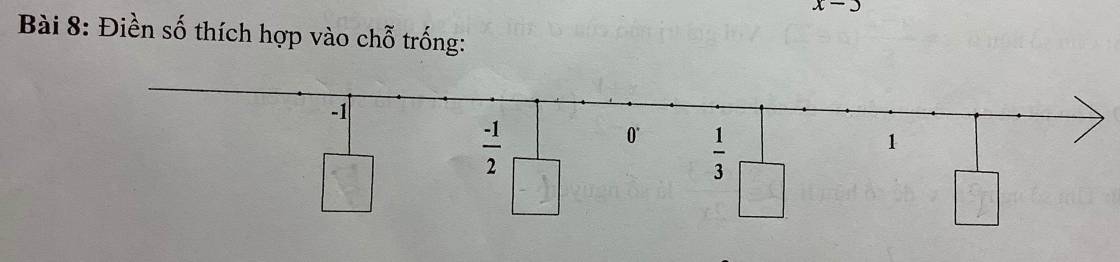
Bài 7:
Vì $Cx\parallel AB$ nên:
$\widehat{C_1}=\widehat{BAC}$ (2 góc so le trong)
$=180^0-\widehat{ABC}-\widehat{ACB}=180^0-75^0-30^0=75^0$
$\widehat{C_2}=180^0-\widehat{ACB}-\widehat{C_1}$
$=180^0-30^0-75^0=75^0$
$\Rightarrow \widehat{C_1}=\widehat{C_2}$
$\Rightarrow Cx$ là tia phân giác của $\widehat{ACy}$
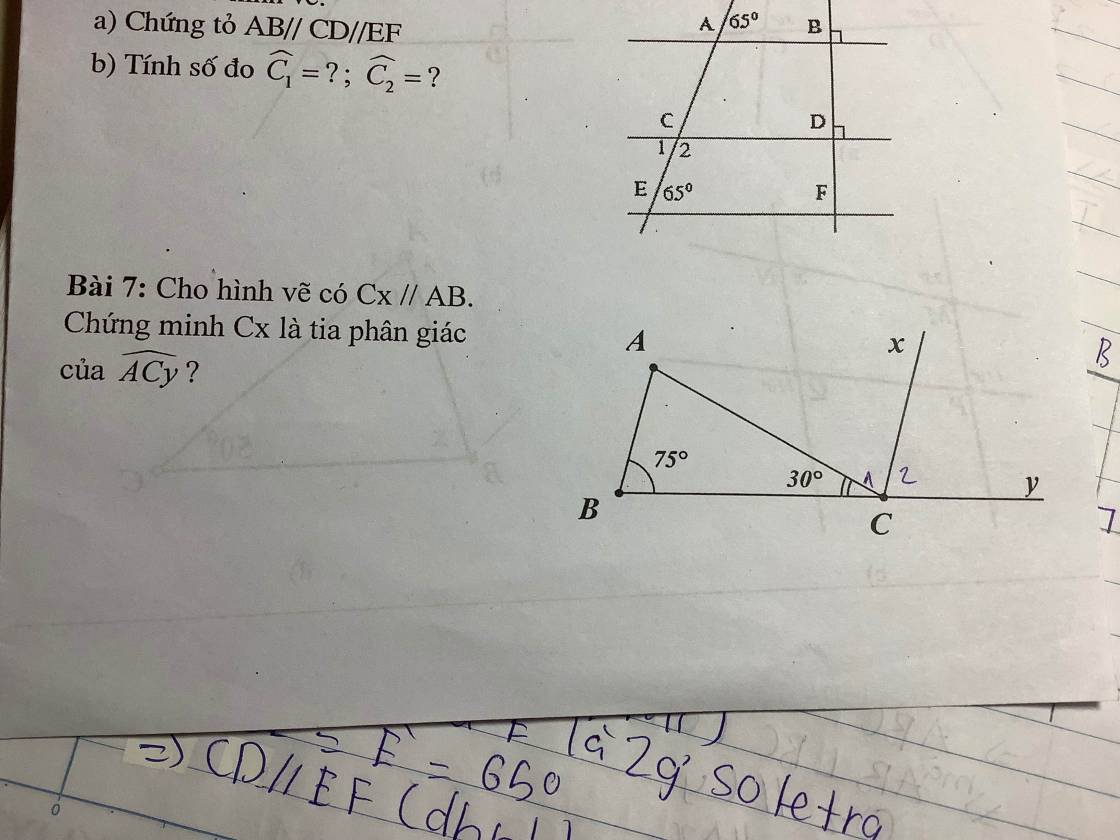
Bài 6:
a. Ta thấy $AB\perp BD, CD\perp BD\Rightarrow AB\parallel CD(1)$
$CD\perp DF, EF\perp DF\Rightarrow CD\parallel EF(2)$
Từ $(1); (2)\Rightarrow AB\parallel CD\parallel EF$
b.
Vì $CD\parallel EF$ nên:
$\widehat{C_1}=\widehat{CEF}=65^0$ (2 góc so le trong)
$\widehat{C_2}=180^0-\widehat{C_1}=180^0-65^0=115^0$








 các bạn giúp mình với mình đang cần gấp nên làm bài nào hay bài đấy
các bạn giúp mình với mình đang cần gấp nên làm bài nào hay bài đấy





