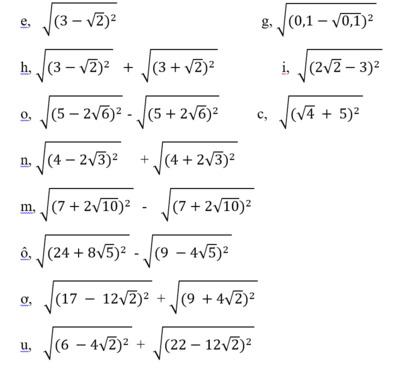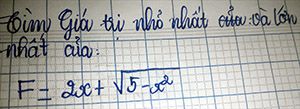Question

Giups mình với ạ. Cảm ơn mng nhiều 

Answer 1

Lời giải:

ĐK: $x>0; x\neq 1$
a.

\(P=\frac{3}{\sqrt{x}}+\left[\frac{x}{\sqrt{x}(\sqrt{x}-1)}+\frac{x+1}{\sqrt{x}}-\frac{1}{\sqrt{x}-1}\right].\frac{\sqrt{x}}{x+\sqrt{x}+1}\)

\(=\frac{3}{\sqrt{x}}+\left[\frac{\sqrt{x}}{\sqrt{x}-1}-\frac{1}{\sqrt{x}-1}+\frac{x+1}{\sqrt{x}}\right].\frac{\sqrt{x}}{x+\sqrt{x}+1}\)

\(=\frac{3}{\sqrt{x}}+\left[\frac{\sqrt{x}-1}{\sqrt{x}-1}+\frac{x+1}{\sqrt{x}}\right].\frac{\sqrt{x}}{x+\sqrt{x}+1}\)

\(=\frac{3}{\sqrt{x}}+(1+\frac{x+1}{\sqrt{x}}).\frac{\sqrt{x}}{x+\sqrt{x}+1}=\frac{3}{\sqrt{x}}+\frac{x+\sqrt{x}+1}{\sqrt{x}}.\frac{\sqrt{x}}{x+\sqrt{x}+1}=\frac{3}{\sqrt{x}}+1\)

b.

$P\geq 10\Leftrightarrow \frac{3}{\sqrt{x}}+1\geq 10$
$\Leftrightarrow \frac{3}{\sqrt{x}}\geq 9$

$\Leftrightarrow \sqrt{x}\leq \frac{1}{3}$

$\Leftrightarrow x\leq \frac{1}{9}$

Kết hợp với ĐKXĐ suy ra $0< x\leq \frac{1}{9}$

c. 

Để $P$ nguyên thì $\frac{3}{\sqrt{x}}$ nguyên.

Với $x$ nguyên, điều này xảy ra khi $\sqrt{x}$ là ước của $3$

$\Leftrightarrow \sqrt{x}\in\left\{1; 3\right\}$

$\Leftrightarrow x\in\left\{1; 9\right\}$ 

Vì $x\neq 1$ nên $x=9$