\(8cosx.sin2x.sin3x\\ =8\left(sin2x.sin3x\right).cosx\\ =8.\dfrac{1}{2}\left[cos\left(2x-3x\right)-cos\left(2x+3x\right)\right].cosx\\ =4.\left(cosx-cos5x\right).cosx\\ =4.cos^2x-4.cos5x.cosx\\ =4.\dfrac{1+cos2x}{2}-4.\dfrac{1}{2}\left[cos4x+cos6x\right]=2\left(1+cos2x\right)-2\left(cos4x+cos6x\right)\\ =2+2.cos2x-2.cos4x-2.cos6x\)
Đúng 3
Bình luận (1)
Các câu hỏi tương tự
Mn giúp em với ạ
Cách chia khối tứ diện thành 6 và 5 phần bằng nhau. Chứng minh thể tích khối chóp bằng thể tích lập phương
cho các số 0,1,2,3,4,5,6,7,8,9 có bao nhiêu số tự nhiên
a)có 3 chữ số khác nhau tạo thành số lẻ
b)có 3 chữ số khác nhau tạo thành số chẵn
c)có 3 chữ số khác nhau nhỏ hơn 540
các bạn giúp mình với
Giải giúp mình bài tập này với. Xin chân thành cảm ơn! Cho tam giác ABC. Dựng bên ngoài ABC tam giác ABD và ACE vuông cân tại B và C. Gọi M là trung điểm DE. Dùng phép biến hình chứng minh tam giác MBC vuông cân. ( Cac ban oi dung phep bien hinh nhe!)
Giúp em với ạ
Một thùng đựng 6 quả cầu trắng và 4 quả cầu đỏ. Chia đôi số quả cầu thành 2 phần bằng nhau. Tìm xác suất cho các biến cố sau đây:
a) Cả hai phần đều có số quả cầu đỏ như nhau
b) Có một phần có 4 quả cầu đỏ
Từ thành phố A có 10 con đường đến thành phố B. Từ thành phố A có 9 con đường đến thành phố C. Từ thành phố B có 6 con đường đến thành phố D. Từ thành phố C có 11 con đường đến thành phố D. Không có con đường nào nối B với C. Hỏi có bao nhiêu cách đi từ A đến D? A. 156 B. 157 C. 159 D. 176
Đọc tiếp
Từ thành phố A có 10 con đường đến thành phố B. Từ thành phố A có 9 con đường đến thành phố C. Từ thành phố B có 6 con đường đến thành phố D. Từ thành phố C có 11 con đường đến thành phố D. Không có con đường nào nối B với C. Hỏi có bao nhiêu cách đi từ A đến D?
A. 156
B. 157
C. 159
D. 176
Các thành phố A, B, C được nối với nhau bởi các con đường như hình vẽ. Hỏi có bao nhiêu cách đi từ thành phố A đến thành phố C mà qua thành phố B chỉ một lần? A. 8 B. 12 C. 6 D. 4
Đọc tiếp
Các thành phố A, B, C được nối với nhau bởi các con đường như hình vẽ. Hỏi có bao nhiêu cách đi từ thành phố A đến thành phố C mà qua thành phố B chỉ một lần?
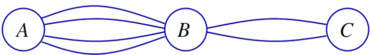
A. 8
B. 12
C. 6
D. 4
Cho hai đường thẳng vuông góc với nhau a và b. có bao nhiêu phép đối xứng trục biến a thành a biến b thành b?
A. Không có phép đối xứng nào
B. có một phép đối xứng trục duy nhất
C. Chỉ có hai phép đối xứng trục
D. Có vô số phép đối xứng trục
Ba số x, y, z theo thứ tự lập thành một cấp số nhân với công bội q khác 1 ; đồng thời các số x ; 2y ; 3z theo thứ tự lập thành một cấp số cộng với công sai khác 0. Tìm giá trị của q. A.
q
1
3
.
B.
q
1
9
.
B.
q
−
1
3
.
D.
q
−
3.
Đọc tiếp
Ba số x, y, z theo thứ tự lập thành một cấp số nhân với công bội q khác 1 ; đồng thời các số x ; 2y ; 3z theo thứ tự lập thành một cấp số cộng với công sai khác 0. Tìm giá trị của q.
A. q = 1 3 .
B. q = 1 9 .
B. q = − 1 3 .
D. q = − 3.
Giúp em với ạ (nếu có thể thì giải thích giúp em với ạ) em cảm ơn nhiều 


Các thành phố A,B,C,D được nối với nhau bằng các con đường như hình vẽ:

b) Số cách đi từ thành phố A đến D, rồi quay lại A là:
A. 24
B. 3600
C. 440
D. 2640









