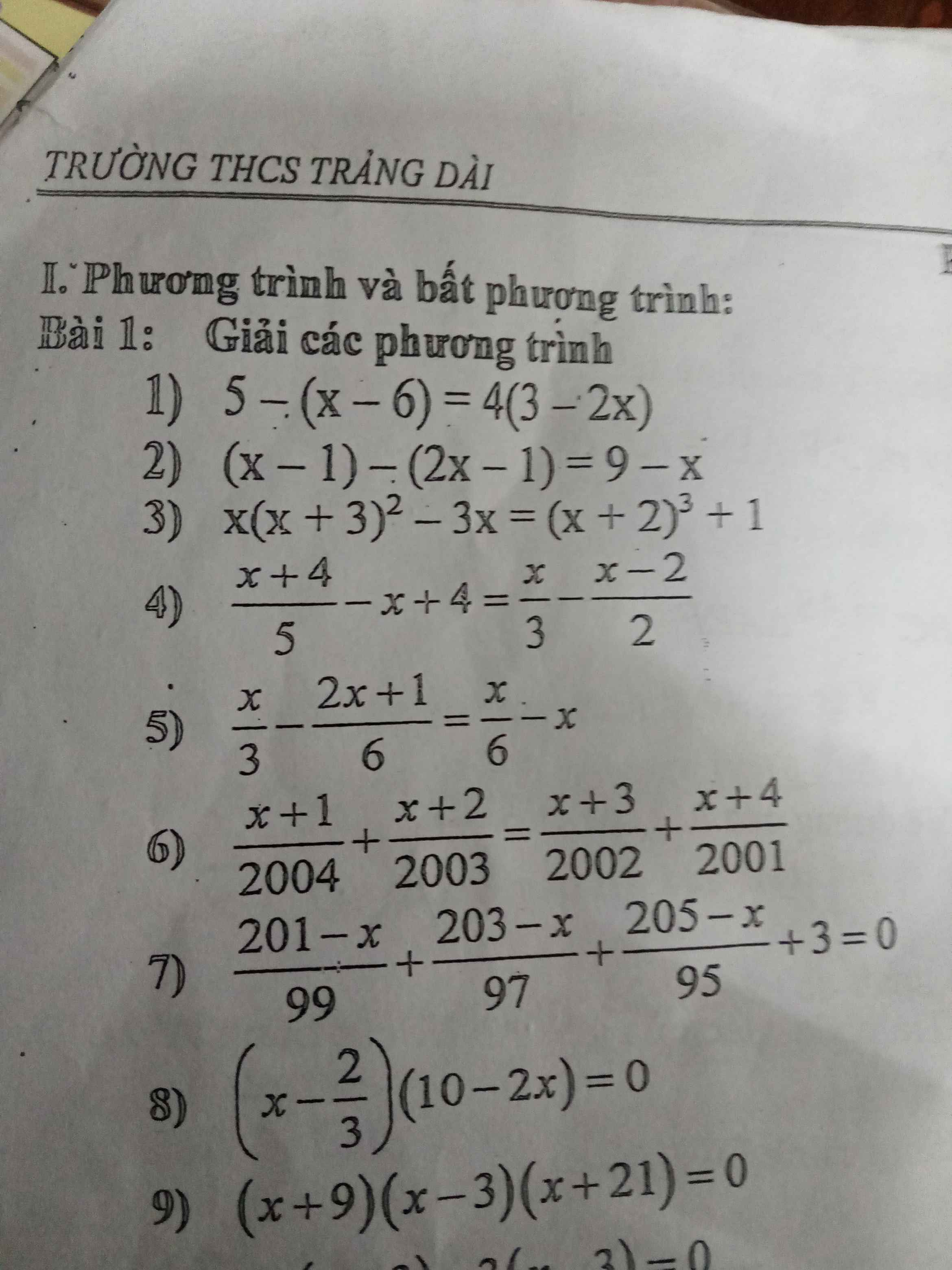`[x+4]/5-x+4=x/3-[x-2]/2`
`<=>[6(x+4)-30(x-4)]/30=[10x-15(x-2)]/30`
`<=>6x+24-30x+120=10x-15x+30`
`<=>6x-30x-10x+15x=30-24-120`
`<=>-19x=-114`
`<=>x=6`
Vậy `S={6}`
\(\dfrac{x+4}{5}-x+4=\dfrac{x}{3}-\dfrac{x-2}{2}\)
\(\Leftrightarrow\dfrac{6\left(x+4\right)+30\left(4-x\right)}{30}=\dfrac{10x-15\left(x-2\right)}{30}\)
\(\Leftrightarrow6\left(x+4\right)+30\left(4-x\right)=10x-15\left(x-2\right)\)
\(\Leftrightarrow6x+24+120-30x=10x-15x+30\)
\(\Leftrightarrow-19x=-114\)
\(\Leftrightarrow x=6\)
Vậy \(S=\left\{6\right\}\)