Đặt \(\dfrac{1}{\sqrt{2x-3y+3}}=a;\dfrac{1}{\sqrt{2x+3y-3}}=b.\left(a;b>0\right).\)
\(HPT.\Rightarrow\) \(\left\{{}\begin{matrix}3a-5b=\dfrac{1}{4}.\\5a+3b=\dfrac{13}{4}.\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=\dfrac{1}{2}.\\b=\dfrac{1}{4}.\end{matrix}\right.\)(TMĐK).
\(\Rightarrow\left\{{}\begin{matrix}\dfrac{1}{\sqrt{2x-3y+3}}=\dfrac{1}{2}.\\\dfrac{1}{\sqrt{2x+3y-3}}=\dfrac{1}{4}.\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\sqrt[]{2x-3y+3}=2.\\\sqrt{2x+3y-3}=4.\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x-3y+3=4.\\2x+3y-3=16.\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x-3y=1.\\2x+3y=19.\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=5.\\y=3.\end{matrix}\right.\)
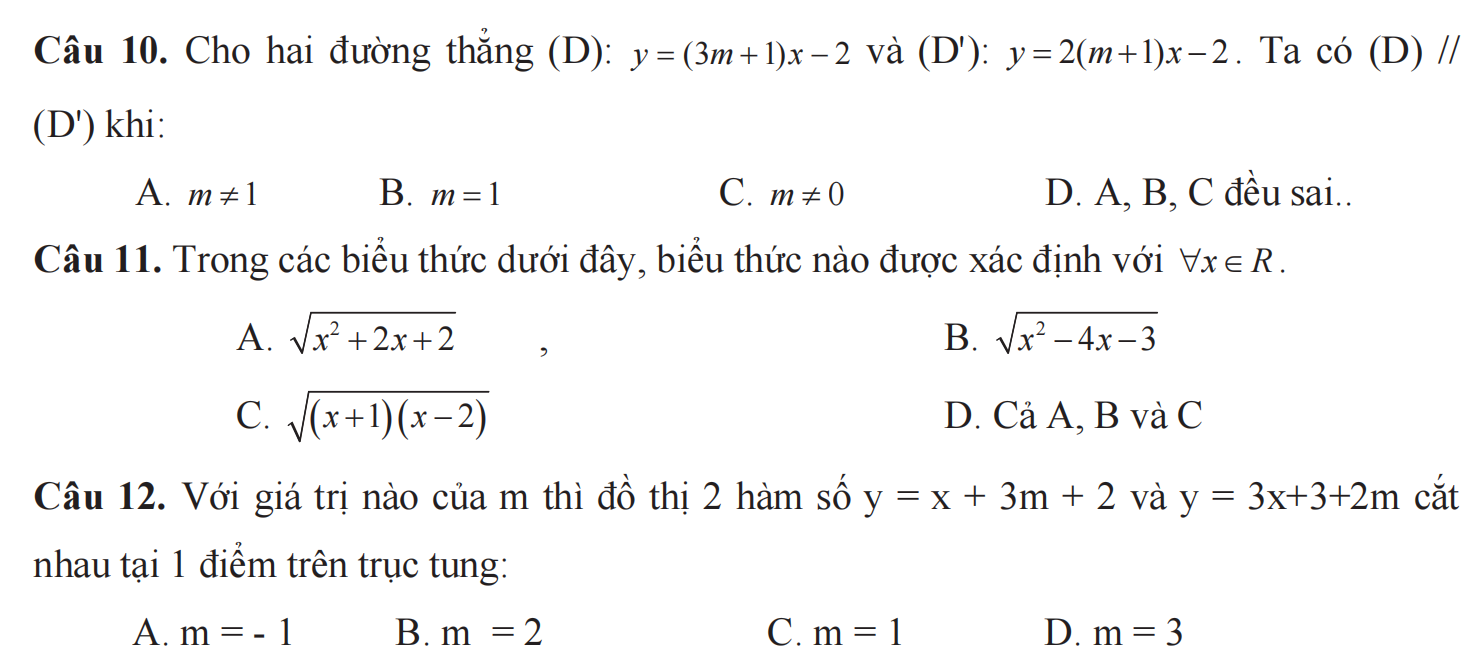
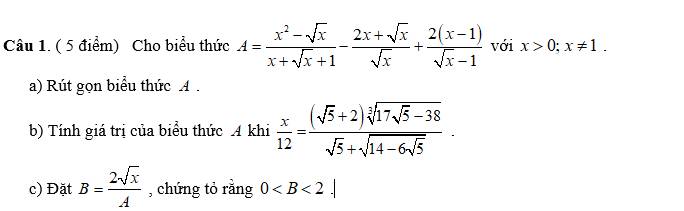
giúp mình với mình đang gấp