Câu 3:
1: ĐKXĐ: x>2
\(\left\{{}\begin{matrix}2\left(y+3\right)-\dfrac{8}{\sqrt{x-2}}=2\\\left(y+3\right)+\dfrac{4}{\sqrt{x-2}}=3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\left(y+3\right)-\dfrac{4}{\sqrt{x-2}}=1\\\left(y+3\right)+\dfrac{4}{\sqrt{x-2}}=3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\left(y+3\right)-\dfrac{4}{\sqrt{x-2}}+\left(y+3\right)+\dfrac{4}{\sqrt{x-2}}=4\\\left(y+3\right)+\dfrac{4}{\sqrt{x-2}}=3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2\left(y+3\right)=4\\\dfrac{4}{\sqrt{x-2}}=3-\left(y+3\right)\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y+3=2\\\dfrac{4}{\sqrt{x-2}}=3-2=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=-1\\x-2=16\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=18\\y=-1\end{matrix}\right.\left(nhận\right)\)
2:
a:
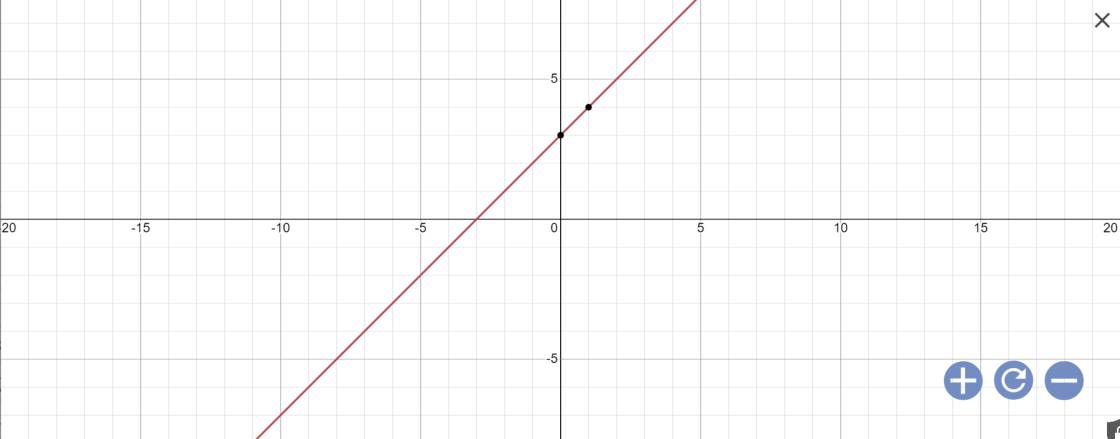
b: Thay x=-2 vào y=x+3, ta được:
\(y=-2+3=1\)
Vậy: A(-2;1)
Thay x=1 vào y=x+3, ta được:
\(y=1+3=4\)
vậy: B(1;4)
c: O(0;0); A(-2;1); B(1;4)
\(OA=\sqrt{\left(-2-0\right)^2+\left(1-0\right)^2}=\sqrt{5}\)
\(OB=\sqrt{\left(1-0\right)^2+\left(4-0\right)^2}=\sqrt{17}\)
\(AB=\sqrt{\left[1-\left(-2\right)\right]^2+\left(4-1\right)^2}=3\sqrt{2}\)
Xét ΔOAB có \(cosAOB=\dfrac{OA^2+OB^2-AB^2}{2\cdot OA\cdot OB}=\dfrac{5+17-18}{2\cdot\sqrt{5}\cdot\sqrt{17}}=\dfrac{2}{\sqrt{85}}\)
=>\(sinAOB=\sqrt{1-cos^2AOB}=\dfrac{9}{\sqrt{85}}\)
Diện tích tam giác AOB là:
\(S_{AOB}=\dfrac{1}{2}\cdot OA\cdot OB\cdot sinAOB\)
\(=\dfrac{1}{2}\cdot\dfrac{9}{\sqrt{85}}\cdot\sqrt{5}\cdot\sqrt{17}=\dfrac{9}{2}\)
Câu 2:
Gọi số tiền ban đầu thầy Tuấn dự định dùng để trả khi mua bút là x( nghìn đồng)
(ĐK: x>0)
Số tiền ban đầu thầy Tuấn dự định dùng để trả khi mua vở là 840-x(nghìn đồng)
Số tiền thực tế thầy Tuấn phải trả khi mua bút là:
\(\left(1-20\%\right)\cdot x=0,8x\left(nghìnđồng\right)\)
Số tiền thực tế thầy Tuấn phải trả khi mua vở là:
\(\left(1-15\%\right)\cdot\left(840-x\right)=0,85\left(840-x\right)\)
Thực tế chỉ phải trả 684000 nên ta có:
0,8x+0,85(840-x)=684
=>-0,05x+714=684
=>-0,05x=-30
=>x=600(nhận)
Vậy: Số tiền dự định phải trả khi mua bút và vở lần lượt là 600 nghìn đồng và 840-600=240 nghìn đồng













 giúp mình câu 4 với câu 2 phần 2 với
giúp mình câu 4 với câu 2 phần 2 với
 Mọi người giúp mình câu 3, câu 4 với. Mình cảm ơn ạ. Mình đang cần gấp lắm!!!
Mọi người giúp mình câu 3, câu 4 với. Mình cảm ơn ạ. Mình đang cần gấp lắm!!!






