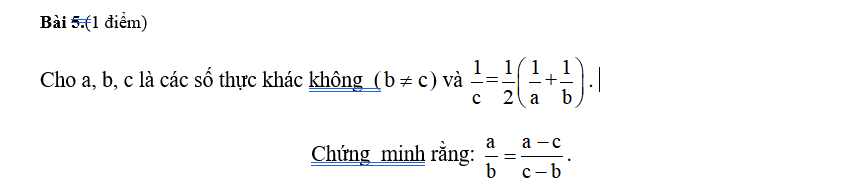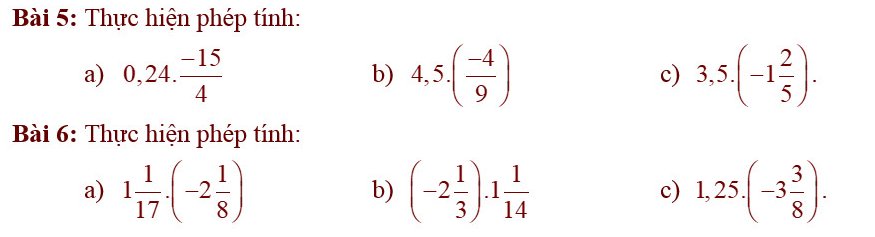f(0)=2023
=>\(a\cdot0^2+b\cdot0+c=2023\)
=>c=2023
=>\(f\left(x\right)=ax^2+bx+2023\)
f(1)=2027
=>\(a\cdot1^2+b\cdot1+2023=2027\)
=>a+b=4
f(-1)=2025
=>\(a\cdot\left(-1\right)^2+b\cdot\left(-1\right)+2023=2025\)
=>a-b=2
mà a+b=4
nên \(a=\dfrac{2+4}{2}=3;b=4-3=1\)
Vậy: \(f\left(x\right)=3x^2+x+2023\)
\(f\left(2\right)=3\cdot2^2+2+2023=12+2025=2037\)






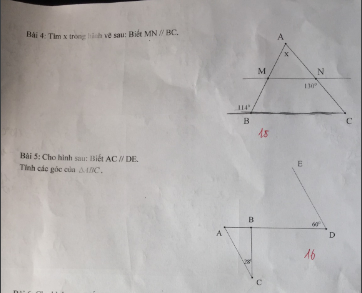 giúp mik với ạ mik cần gấp. GIẢI CỤ THỂ GIÚP MIK vs ạ
giúp mik với ạ mik cần gấp. GIẢI CỤ THỂ GIÚP MIK vs ạ