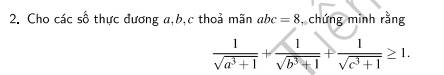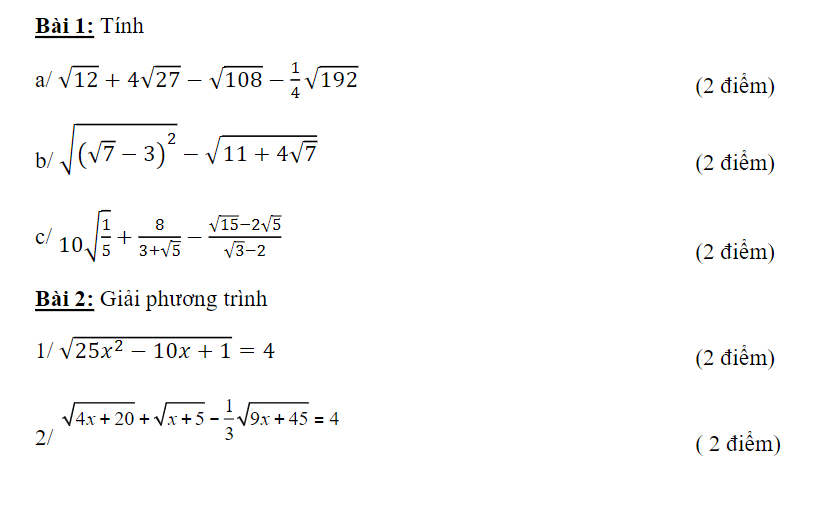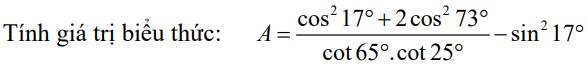1: \(\left\{{}\begin{matrix}4x+5y=3\\x-2y=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}4x+5y=3\\4x-8y=20\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}13y=-17\\x-2y=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=-\dfrac{17}{13}\\x=5+2y=5-\dfrac{34}{13}=\dfrac{31}{13}\end{matrix}\right.\)
2: \(\left\{{}\begin{matrix}7x-2y=1\\3x+y=6\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}7x-2y=1\\6x+2y=12\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}13x=13\\3x+y=6\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=1\\y=6-3x=6-3=3\end{matrix}\right.\)
3: \(\left\{{}\begin{matrix}10x-9y=8\\15x+21y=0,5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}30x-27y=24\\30x+42y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-69y=23\\10x-9y=8\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=-\dfrac{1}{3}\\10x=9y+8=-3+8=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=-\dfrac{1}{3}\\x=\dfrac{1}{2}\end{matrix}\right.\)
4: \(\left\{{}\begin{matrix}1,7x-4y=3,8\\2,1x+5y=0,4\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}8,5x-20y=19\\8,4x+20y=1,6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}16,9x=19,6\\1,7x-4y=3,8\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=\dfrac{196}{169}\\4y=1,7x-3,8=-\dfrac{309}{169}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=\dfrac{196}{169}\\y=-\dfrac{309}{676}\end{matrix}\right.\)
6:
\(\left\{{}\begin{matrix}2x-11y=-7\\10x+11y=31\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}12x=24\\2x-11y=-7\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=2\\11y=2x+7=11\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\)

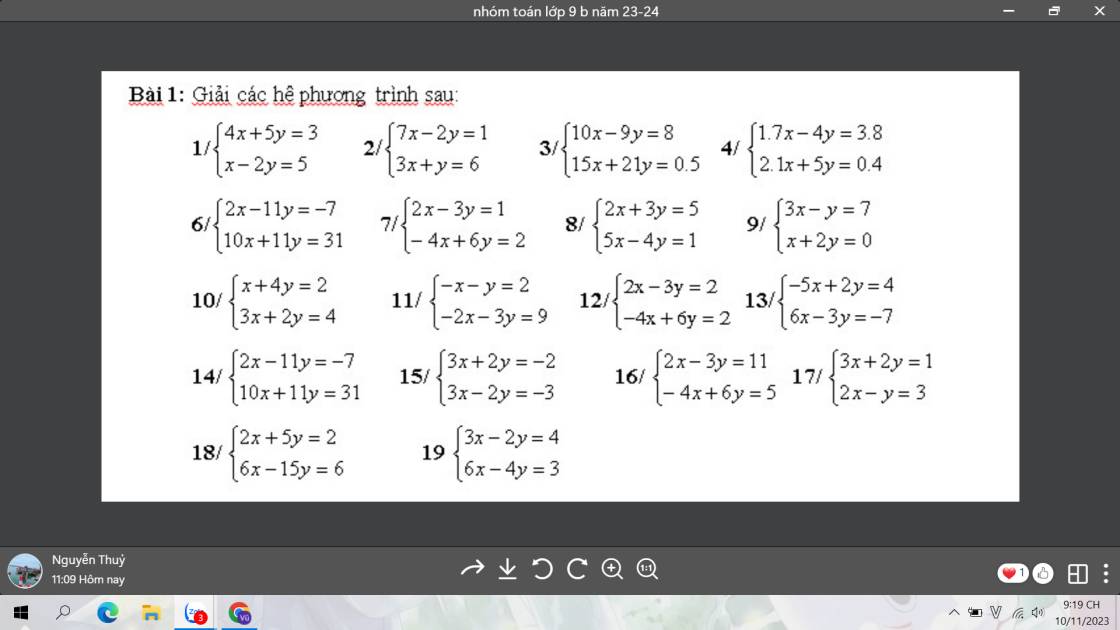 giúp mik với
giúp mik với 



 Mn giúp mik với,bh mik đang cần gấp lắm 19h30 mik phải có bài r nên mn giúp mik với
Mn giúp mik với,bh mik đang cần gấp lắm 19h30 mik phải có bài r nên mn giúp mik với 





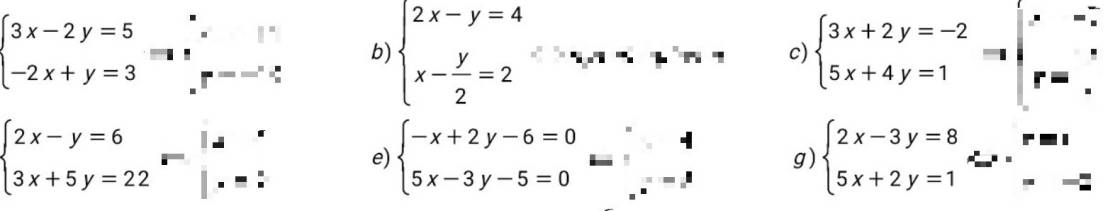
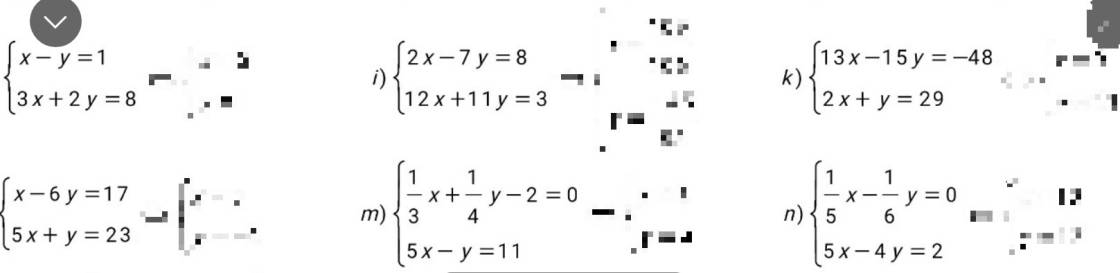 giúp mik với mik đg cần gấp
giúp mik với mik đg cần gấp