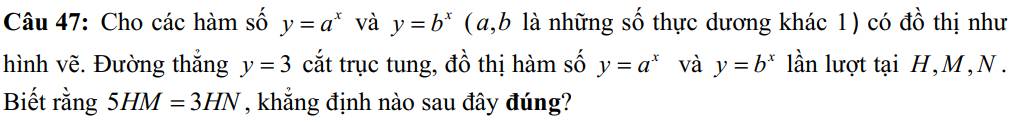Question

Giúp em với ạ !!!!!!

Chọn và giải thích tại sao lại chọn đáp án đó ạ. Em cảm ơn ạ 

Answer 1

Câu 102:

ĐKXĐ: $-1\leq x\leq 3$
\(\log_{\frac{1}{2}}(3-x)-\log_2(x+1)\geq 0\\ \Leftrightarrow \log_{\frac{1}{2}}(2).\log_2(3-x)-\log_2(x+1)\geq 0\\ \Leftrightarrow -\log_2(3-x)-\log_2(x+1)\geq 0\\ \Leftrightarrow \log_2(3-x)+\log_2(x+1)\leq 0\\ \Leftrightarrow \log_2[(3-x)(x+1)]\leq 0\\ \Leftrightarrow 0<(3-x)(x+1)\leq 1\)

Từ $(3-x)(x+1)\leq 1$

$\Leftrightarrow (x-3)(x+1)+1\geq 0$

$\Leftrightarrow x^2-2x-2\geq 0$

$\Leftrightarrow (x-1)^2\geq 3$

$\Leftrightarrow x-1\geq \sqrt{3}$ hoặc $x-1\leq -\sqrt{3}$

$\Leftrightarrow x\geq 1+\sqrt{3}$ hoặc $x\leq 1-\sqrt{3}$

Kết hợp với đkxđ suy ra $x\in [1+\sqrt{3};3)\cup (-1; 1-\sqrt{3}]$

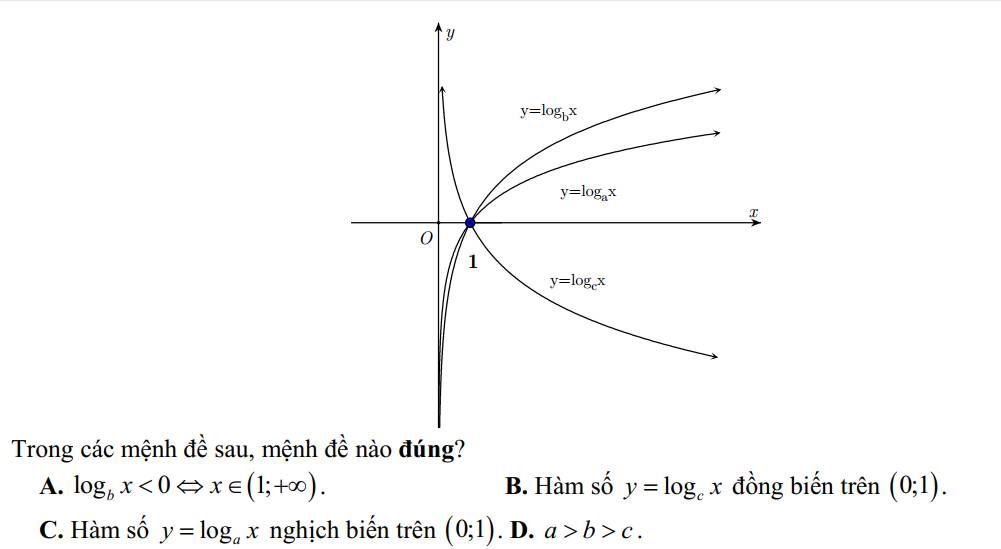
Đáp án A

Answer 2

Câu 106:

\(\text{BPT} \Leftrightarrow \log_{\sqrt{3}}(3).\log_3(\sqrt{x})+\log_{\frac{1}{3}}(3).\log_3(x^3)+\log_3(3x^4)>3\)

\(\Leftrightarrow 2\log_3(\sqrt{x})-\log_3(x^3)+\log_3(3x^4)>3\\ \Leftrightarrow \log_3(x)-\log_3(x^3)+\log_3(x^4)+1>3\\ \Leftrightarrow \log_3(\frac{x.x^4}{x^3})>2\\ \Leftrightarrow \log_3(x^2)>2\\ \Leftrightarrow 2\log_3(x)>2\\ \Leftrightarrow \log_3(x)>1\Leftrightarrow x>3\)

Đáp án B.

Answer 3

Lời giải:

ĐK: $x>0$

Đặt $\log_{\frac{x}{8}}(2)=a\Rightarrow 2=(\frac{x}{8})^a$

$\Rightarrow 2^{3a+1}=x^a$

$\Rightarrow (3a+1)\log_x2=a$

$\Rightarrow \log_x2=\frac{a}{3a+1}$ ($3a+1\neq 0$)

Khi đó:

$\log_x2\leq \log_{\frac{x}{8}}2$

$\Leftrightarrow \frac{a}{3a+1}\leq a$

$\Leftrightarrow \frac{-3a^2}{3a+1}\leq 0$

$\Leftrightarrow 3a+1>0$

$\Leftrightarrow a> \frac{-1}{3}$

Khi đó:

$\log_{\frac{x}{8}}(2)> \frac{-1}{3}(*)$

Nếu $\frac{x}{8}>1$ thì $(*)\Leftrightarrow 2> (\frac{x}{8})^{\frac{-1}{3}}$

$\Leftrightarrow 2^3> \frac{8}{x}$

$\Rightarrow x> 1$

Mà: $\frac{x}{8}>1\Rightarrow x>8$. Mà $x$ nguyên nên: $x\in \left\{9; 10; .....; 2024\right\}$ => 2016 giá trị

Nếu $0< \frac{x}{8}<1$ thì $(*)\Leftrightarrow 2<(\frac{x}{8})^{\frac{-1}{3}}$

$\Rightarrow 8< \frac{8}{x}$

$\Rightarrow x< 1$

Mà $x>0$ nên không có giá trị nguyên nào tm.

Vậy...........

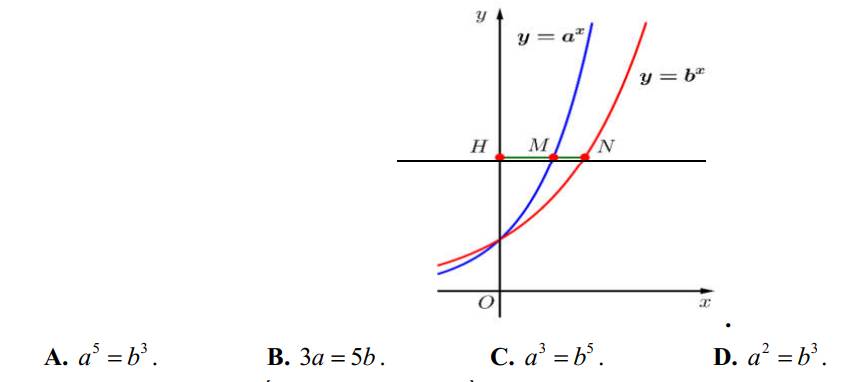
Đáp án C

Answer 4

Câu 108:

$\log_{\sqrt{3}}(x^2+x)> \log_{\sqrt{3}}(-2x+4)$

$\Leftrightarrow x^2+x> -2x+4$

$\Leftrightarrow x^2+3x-4>0$

$\Leftrightarrow (x-1)(x+4)>0$

$\Leftrightarrow x> 1$ hoặc $x< -4$ (1)

Mà $-2x+4>0\Rightarrow x< 2$ (2)

$x^2+x>0\Leftrightarrow x(x+1)>0\Leftrightarrow x>0$ hoặc $x<-1$ (3)

Kết hợp (1); (2); (3) suy ra $x\in (1;2)\cup (-\infty; -4)$

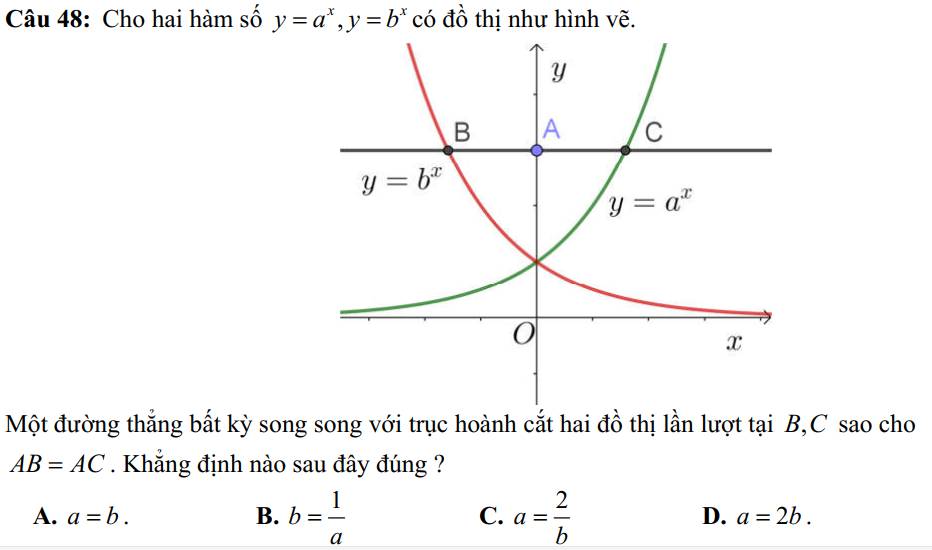
Đáp án B.