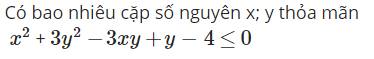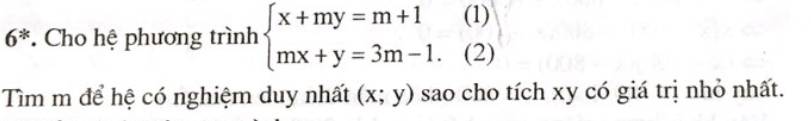11.
\(\Delta=\left(m+3\right)^2-4\left(m-5\right)=m^2+2m+29=\left(m+1\right)^2+28>0;\forall m\)
\(\Rightarrow\) Phương trình luôn có 2 nghiệm pb với mọi m
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=-m-3\\x_1x_2=m-5\end{matrix}\right.\)
\(\left|x_1\right|+\left|x_2\right|=8\)
\(\Leftrightarrow x_1^2+x_2^2+2\left|x_1x_2\right|=64\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2+2\left|x_1x_2\right|=64\)
\(\Leftrightarrow\left(-m-3\right)^2-2\left(m-5\right)+2\left|m-5\right|=64\) (1)
TH1: \(m\ge5\Rightarrow\left|m-5\right|=m-5\)
(1) \(\Leftrightarrow\left(m+3\right)^2=64\Rightarrow\left[{}\begin{matrix}m=5\left(tm\right)\\m=-11< 5\left(ktm\right)\end{matrix}\right.\)
TH2: \(m< 5\Rightarrow\left|m-5\right|=5-m\)
\(\left(1\right)\Leftrightarrow\left(m+3\right)^2+2\left(m-5\right)-2\left(5-m\right)=64\)
\(\Leftrightarrow m^2+10m-75=0\Rightarrow\left[{}\begin{matrix}m=5\left(ktm\right)\\m=-15\left(tm\right)\\\end{matrix}\right.\)
Vậy \(m=\left\{5;-15\right\}\)
13.
\(\Delta=\left(2m+5\right)^2-4m^2=20m+25\)
Pt có 2 nghiệm pb khi và chỉ khi:
\(20m+25>0\Rightarrow m>-\dfrac{5}{4}\)
Khi đó theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2m+5\\x_1x_2=m^2\end{matrix}\right.\)
Do \(x_1;x_2\) là độ dài 2 cạnh góc vuông có độ dài cạnh huyền bằng \(\sqrt{17}\Rightarrow x_1;x_2>0\) và theo định lý Pitago:
\(x_1^2+x_2^2=17\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=17\)
\(\Leftrightarrow\left(2m+5\right)^2-2m^2=17\)
\(\Leftrightarrow2m^2+20m+8=0\Rightarrow\left[{}\begin{matrix}m=-5+\sqrt{21}\left(tm\right)\\m=-5-\sqrt{21}< -\dfrac{5}{4}\left(ktm\right)\end{matrix}\right.\)
12.
\(\Delta'=1-\left(2m-5\right)=6-2m\)
Phương trình có 2 nghiệm pb khi và chỉ khi:
\(\Delta'=6-2m>0\)
\(\Rightarrow m< 3\)
Khi đó theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\\x_1x_2=2m-5\end{matrix}\right.\)
Để \(x_2=5x_1\Rightarrow x_2=5\left(2-x_1\right)\)
\(\Leftrightarrow6x_2=10\Rightarrow x_2=\dfrac{5}{3}\)
\(\Rightarrow x_1=2-x_2=\dfrac{1}{3}\)
Thế vào \(x_1x_2=2m-5\)
\(\Rightarrow2m-5=\dfrac{5}{9}\)
\(\Rightarrow m=\dfrac{25}{9}\) (thỏa mãn)
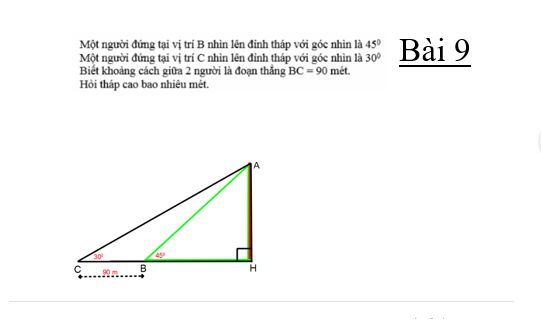giúp em với ạ. Em cảm ơn ạ
