ĐKXĐ: x<>1 và y>-2
\(\left\{{}\begin{matrix}\dfrac{2}{x-1}-\dfrac{3}{\sqrt{y+2}}=-1\\\dfrac{1}{x-1}+\dfrac{4}{\sqrt{y+2}}=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\dfrac{2}{x-1}-\dfrac{3}{\sqrt{y+2}}=-1\\\dfrac{2}{x-1}+\dfrac{8}{\sqrt{y+2}}=10\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-\dfrac{11}{\sqrt{y+2}}=-11\\\dfrac{2}{x-1}-\dfrac{3}{\sqrt{y+2}}=-1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\sqrt{y+2}=1\\\dfrac{2}{x-1}=-1+3=2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x-1=1\\y+2=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=2\\y=-1\end{matrix}\right.\left(nhận\right)\)
đk x khác 1 ; y > -2
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{2}{x-1}-\dfrac{3}{\sqrt{y+2}}=-1\\\dfrac{2}{x-1}+\dfrac{8}{\sqrt{y+2}}=10\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{11}{\sqrt{y+2}}=11\\\dfrac{2}{x-1}-\dfrac{3}{\sqrt{y+2}}=-1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y=-1\\\dfrac{2}{x-1}-3=-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=-1\\x=2\end{matrix}\right.\)

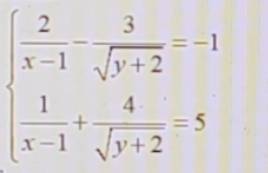 giải hệ phương trình
giải hệ phương trình










